Advertisements
Advertisements
प्रश्न
Two dice are thrown. Find the probability of the following events:
Event A : The product of the numbers on their upper faces is 10.
Event B : The sum of the numbers on their upper faces is 10
उत्तर
Since two dice are thrown,
S ≡ {(1,1) (1,2) (1,3) (1,4) (1,5) (1,6)
(2,1) (2,2) (2,3) (2,4) (2,5) (2,6)
(3,1) (3,2) (3,3) (3,4) (3,5) (3,6)
(4,1) (4,2) (4,3) (4,4) (4,5) (4,6)
(5,1) (5,2) (5,3) (5,4) (5,5) (5,6)
(6,1) (6,2) (6,3) (6,4) (6,5) (6,6)}
∴ n(S) = 36
(A) Let A ≡ Event of getting the product of the numbers is 10.
A ≡ {(2,5) (5,2)}
∴ n(A) = 2
∴ P(A) = `("n"("A"))/("n"("S")) = 2/36 = 1/18`
∴ P(A) = `1/18`
(B) Let B ≡ Event of getting the sum of the numbers is a multiple of 9.
∴ B ≡ {(3,6) (4,5) (5,4) (6,3)}
∴ n(B) = 4
∴ P(B) = `("n"("B"))/("n"("S")) = 4/36`
∴ P(B) = `1/9`
APPEARS IN
संबंधित प्रश्न
All the black face cards are removed from a pack of 52 playing cards. The remaining cards are well shuffled and then a card is drawn at random. Find the probability of getting a:
(i) face card
(ii) red card
(iii) black card
(iv) king
Cards numbered from 11 to 60 are kept in a box. If a card is drawn at random from the box, find the probability that the number on the drawn card is a perfect square number.
A bag contains cards numbered from 1 to 49. A card is drawn from the bag at random, after mixing the cards thoroughly. Find the probability that the number on the drawn card is a perfect square.
In fig. 7 is shown a disc on which a player spins an arrow twice. The fraction `a/b` is formed, where 'a' is the number of sector on which arrow stops on the first spin and 'b' is the number of the sector in which the arrow stops on second spin. On each spin, each sector has equal chance of selection by the arrow. Find the probability that the fraction `a/b>1.`

Joseph kept 26 cards in a cap, bearing one English alphabet on each card. One card is drawn at random. What is the probability that the card drawn is a vowel card ?
In a class of 42 students in Model High School, 3 students use spectacles.
Fill in the following boxes to find the probability of a students selected
at random is wearing sepctacles.
The total number of students in the class is 42.
∴n(S) = ...... ,
Let the event, a student uses spectacles, be A.
∴ n(A) = ...........
∴ P(A) = ............ ∴ P(A) = ............
Cards numbered 1 to 30 are put in a bag. A card is drawn at random from this bag. Find the probability that the number on the drawn card is a prime number greater than 7
A coin is tossed once. What is the probability of getting a tail?
Two different dice are rolled simultaneously. Find the probability that the sum of the numbers on the two dice is 10.
What is the probability that an ordinary year has 53 Mondays?
In a certain race there are three boys A, B, C. The winning probability of A is twice than B and the winning probability of B is twice than C. If P(A) + P(B) + P(C) = 1, then find the probabilities of their winning.
A die is thrown once. Find the probability of getting a number which
lies between 2 and 6.
A bag contains 15 balls, out of which some are white and the others are black. If the probability of drawing a black ball at random from the bag is `2/3`, then find how many white balls are there in the bag.
A die is thrown once. Find the probability of getting:
a prime number
There are 24 balls in a pot. If 3 of them are Red, 5 of them are Blue and the remaining are Green then, what is the probability of picking out a Red ball?
A coin is tossed 12 times and the outcomes are observed as shown below:
The chance of occurrence of Head is
A dice is rolled once. What is the probability that the number on top will be a multiple of 3
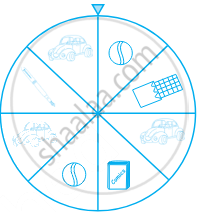
At a Birthday Party, the children spin a wheel to get a gift. Find the probability of getting a toy car
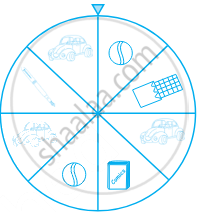
At a Birthday Party, the children spin a wheel to get a gift. Find the probability of any toy except a chocolate
Sonia picks up a card from the given cards.

Calculate the probability of getting a G card
