Advertisements
Advertisements
प्रश्न
Use a suitable identity to get the following products.
(2y + 5) (2y + 5)
उत्तर
The product will be as follow
(2y + 5) (2y + 5) = (2y + 5)2
= (2y)2 + 2(2y) (5) + (5)2 [(a + b)2 = a2 + 2ab + b2]
= 4y2 + 20y + 25
APPEARS IN
संबंधित प्रश्न
Find the following squares by suing the identities.
(6x2 − 5y)2
Using identities, evaluate 1.05 × 9.5
Using (x + a) (x + b) = x2 + (a + b) x + ab, find 5.1 × 5.2
Use an expansion formula to find the value.
(102)2
`(("a" + "b")("a"^3 - "b"^3))/(("a"^2 - "b"^2))` = ___________
Expand the following square, using suitable identities
(2x + 5)2
(a + b)2 – 2ab = ______ + ______.
Factorise `x^2 + 1/x^2 + 2 - 3x - 3/x`.
Take suitable number of cards given in the adjoining diagram [G(x × x) representing x2, R(x × 1) representing x and Y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles:
- 2x2 + 6x + 4
- x2 + 4x + 4.
Factorise 2x2 + 6x + 4 by using the figure.
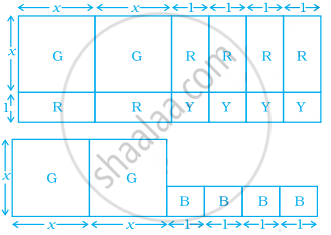
Calculate the area of figure.
Take suitable number of cards given in the adjoining diagram [G(x × x) representing x2, R(x × 1) representing x and Y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles: x2 + 4x + 4. Factorise 2x2 + 6x + 4 by using the figure.
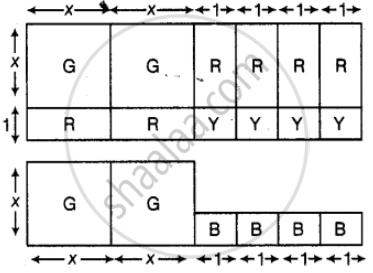
Calculate the area of figure.
