Advertisements
Advertisements
Question
A bucket made of aluminum sheet is of height 20cm and its upper and lower ends are of radius 25cm an 10cm, find cost of making bucket if the aluminum sheet costs Rs 70 per
100 cm2
Solution
Given that,
The radii of the upper and lower circles of the bucket are r1 = 25 cm and r2= 10 cm respectively.
The height of the bucket = 20 cm
The slant height of the bucket
`l=sqrt(h^2+(r_1-r_2)^2`
`=sqrt(20^2+(25-10)^2`
`=sqrt(400+225)`
`=sqrt(625)`
= 25 cm
The surface area of the used aluminium sheet to make the bucket
`S=pi(r_1+r_2)xxl+pir_2^2`
= 3.14(25+10) x 25 + 3.14 x 10 x 10
= 3.14 x 35 x 25 + 3.14 x 100
= 2747.5 + 314
= 3061.5 cm
It is given that the cost of aluminium sheet is Rs 70 per 100 cm2
therefore, the total cost of making the bucket
`Rs 3061.5/100xx70`
Rs 2143.05
Hence, the total cost of making the bucket is Rs 2143.05.
APPEARS IN
RELATED QUESTIONS
Due to sudden floods, some welfare associations jointly requested the government to get 100 tents fixed immediately and offered to contribute 50% of the cost. If the lower part of each tent is of the form of a cylinder of diameter 4.2 m and height 4 m with the conical upper part of same diameter but of height 2.8 m, and the canvas to be used costs Rs. 100 per sq. m, find the amount, the associations will have to pay. What values are shown by these associations? [Use π=22/7]
504 cones, each of diameter 3.5 cm and height 3 cm, are melted and recast into a metallic sphere. Find the diameter of the sphere and hence find its surface area.
[Use π=22/7]
From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2
[use `pi = 22/7`]
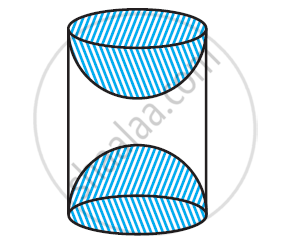
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in given figure. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the total surface area of the article.
[Use `pi = 22/7`]
From a solid right circular cylinder of height 2.4 cm and radius 0.7 cm, a right circular cone of same height and same radius is cut out. Find the total surface area of the remaining solid.
A cylindrical tub, whose diameter is 12 cm and height 15 cm is full of ice-cream. The whole ice-cream is to be divided into 10 children in equal ice-cream cones, with conical base surmounted by hemispherical top. If the height of conical portion is twice the diameter of base, find the diameter of conical part of ice-cream cone ?
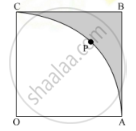
In Fig. 6, OABC is a square of side 7 cm. If OAPC is a quadrant of a circle with centre O, then find the area of the shaded region. `[\text\ User=22/7]`
A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter 4 \[\frac{2}{3}\] cm and height 3 cm. Find the number of cones so formed.
How many cubes of 10 cm edge can be put in a cubical box of 1 m edge?
Statement A (Assertion): Total Surface area of the top is the sum of the curved surface area of the hemisphere and the curved surface area of the cone.
Statement R( Reason): Top is obtained by joining the plane surfaces of the hemisphere and cone together.

