Advertisements
Advertisements
Question
A capsule is in the shape of a cylinder with two hemispheres stuck to its ends. If the length of the entire capsule is 12 mm and the diameter of the capsule is 3 mm, how much medicine it can hold?
Solution
Radius of a hemisphere = Radius of a Cylinder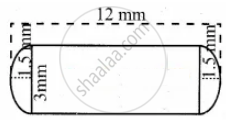
r = `3/2` mm = 1.5 mm
Height of the cylinderical portion
= 12 mm – (1.5 mm + 1.5 mm)
= (12 – 3) mm
= 9 mm
Volume of the capsule
= `pi"r"^2"h" + 2 xx 2/3 pi"r"^3`
= `pi"r"^2 ("h" + 4/3"r")`
= `22/7 xx 1.5 xx 1.5 (9 + 4/3 xx 1.5)`
= `22/7 xx 1.5 xx 1.5(9 + 2)`
= `22/7 xx 1.5 xx 1.5 xx 11 "mm"^3`
= 77.8 cu.mm
Volume of the capsule = 77.8 cu.mm
APPEARS IN
RELATED QUESTIONS
Water flows at the rate of 15 m per minute through a cylindrical pipe, having the diameter 20 mm. How much time will it take to fill a conical vessel of base diameter 40 cm and depth 45 cm?
A farmer connects a pipe of internal diameter 20 cm from a canal into a cylindrical tank which is 10 m in diameter and 2 m deep. If the water flows through the pipe at the rate of 4 km per hour, in how much time will the tank be filled completely?
A cylinder and a cone have equal bases. The height of the cylinder is 3 cm and the area of its base is 100 cm2. The cone is placed upon the cylinder. The volume of the solid figure so formed is 500 cm3. Find the total height of the figure.

In the given figure shows a toy. Its lower part is a hemisphere and the upper part is a cone. Find the volume and surface area of the toy from the measures shown in the figure (\[\pi = 3 . 14\])
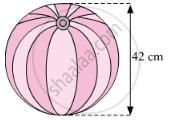
Find the surface area and the volume of a beach ball shown in the figure.
A cylindrical bucket of diameter 28 cm and a height of 20 cm was full of sand. When the sand in the bucket was poured on the ground, the sand got converted into a shape of a cone. If the height of the cone was 14 cm, what was the base area of the cone?
A tin maker converts a cubical metallic box into 10 cylindrical tins. The side of the cube is 50 cm and the radius of the cylinder is 7 cm. Find the height of each cylinder so made, if the wastage of 12% is incurred in the process `(pi = 22/7)`
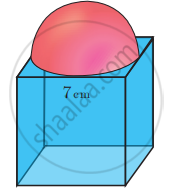
As shown in the figure a cubical block of side 7 cm is surmounted by a hemisphere. Find the surface area of the solid.
A right circular cylinder just encloses a sphere of radius r units. Calculate the ratio of the areas of the sphere and cylinder
A cylinder and a cone have equal bases. The height of the cylinder is 2 cm and the area of its base is 64 cm2. The cone is placed upon the cylinder volume of the solid figure so formed is 400 cm3. Find the total height of the figure.