Advertisements
Advertisements
Question
A closed surface in vacuum encloses charges –q and +3q. The total electric flux emerging out of the surface is :
Options
Zero
`(2q)/∈_0`
`(3q)/∈_0`
`(4q)/∈_0`
Solution
`(2q)/∈_0`
`phi = q_(enc)/∈_0 = (+3q - q)/∈_0 = (2q)/∈_0`
APPEARS IN
RELATED QUESTIONS
A point charge causes an electric flux of −1.0 × 103 Nm2/C to pass through a spherical Gaussian surface of 10.0 cm radius centred on the charge.
- If the radius of the Gaussian surface were doubled, how much flux would pass through the surface?
- What is the value of the point charge?
If the point charge is now moved to a distance 'd' from the centre of the square and the side of the square is doubled, explain how the electric flux will be affected.
Draw a graph to show the variation of E with perpendicular distance r from the line of charge.
Find the work done in bringing a charge q from perpendicular distance r1 to r2 (r2 > r1)
Which statement is true for Gauss law -
If the ratio of radii of two wires of same material is 3 : 1 and ratio of their lengths is 5 : 1, then the ratio of the normal forces that will produce the same extension in the length of two wires is:
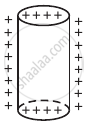
Sketch the electric field lines for a uniformly charged hollow cylinder shown in figure.
Consider a sphere of radius R with charge density distributed as
ρ(r) = kr for r ≤ R
= 0 for r > R
- Find the electric field at all points r.
- Suppose the total charge on the sphere is 2e where e is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.
An infinitely long positively charged straight wire has a linear charge density λ. An electron is revolving in a circle with a constant speed v such that the wire passes through the centre, and is perpendicular to the plane, of the circle. Find the kinetic energy of the electron in terms of the magnitudes of its charge and linear charge density λ on the wire.
