Question

A father tells his daughter, “ Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be.” Represent this situation algebraically and graphically.

Answer 1

Let the present age of father be x years and that of daughter = y years

Seven years ago father’s age = (x – 7) years

Seven years ago daughter’s age = (y – 7) years

According to the problem

(x – 7) = 7(y – 7) or x – 7y = – 42 ….(1)

After 3 years father’s age = (x + 3) years

After 3 years daughter’s age = (y + 3) years

According to the condition given in the question

x + 3 = 3(y + 3) or x – 3y = 6 ….(2)

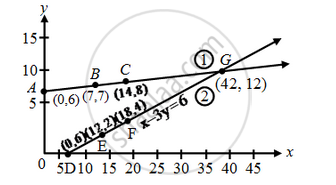
`x – 7y = –42 ⇒ y=\frac { x+42 }{ 7 }`

x	0	7	14
y	6	7	8
Points	A	B	C

`x – 3y = 6   ⇒   y=\frac { x-6 }{ 3 }`

x	6	12	18
y	0	2	4
Points	D	E	F

Plot the points A(0, 6), B(7, 7), C(14, 8) and join them to get a straight line ABC. Similarly plot the points D(6, 0), E(12, 2) and F(18,4) and join them to get a straight line DEF.