Advertisements
Advertisements
Question
A man starts his morning walk at a point A reaches two points B and C and finally back to A such that ∠A = 60° and ∠B = 45°, AC = 4 km in the ∆ABC. Find the total distance he covered during his morning walk
Solution
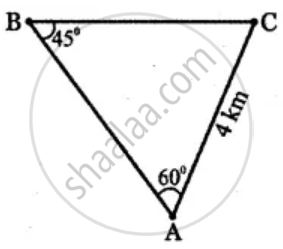
Given In ∆ABC
AC = 4 km
∠A = 60°,
∠B = 45°
∠C = 180° – (60° + 45°)
∴ ∠C = 180° – 105° = 75°
Using sine formula
`"BC"/sin"A" = "AC"/sin"B" = "AB"/sin "C"`
`"BC"/(sin 60^circ) = 4/(sin 45^circ) = "AB"/(sin 75^circ)`
`"BC"/(sin 60^circ) = 4/(sin 45^circ) = "AB"/(sin 75^circ)`
`"BC"/(sin 60^circ) = 4/(sin 45^circ)`
BC = `4/(sin 45^circ) xx sin 60^circ`
BC = `4/(1/sqrt(2)) xx sqrt(3)/2`
BC = `2sqrt(3) * sqrt(2)`
= `2sqrt(6)` km
`4/(sin 45^circ) = "AB"/(sin 75^circ)`
`4/(1/sqrt(2)) = "AB"/(sin(45^circ + 30^circ))`
`4sqrt(2) = "AB"/(sin 4^circ cos30^circ + cos 45^circ sin 30^circ)`
`4sqrt(2) = "AB"/(1/sqrt() * sqrt(3)/2 + 1/sqrt(2) * 1/2)`
`4sqrt(2) = "AB"/((sqrt(3) + 1)/(2sqrt(2))`
`4sqrt(2) = (2sqrt(2)"AB")/(sqrt(3) + 1)`
2 = `"AB"/(sqrt(3) + 1)`
AB = `2(sqrt(3) + 1)` km.
Total distance of morning walk
= AB + BC + AC
= `2(sqrt(3) + 1) + 2sqrt(6) + 4`
= `2sqrt(3) + 2 + 2sqrt(6) + 4`
= `2sqrt(3) + 6 + 2sqrt(6)`
= `6 + 2sqrt(6) + 2sqrt(3)` km.
APPEARS IN
RELATED QUESTIONS
Determine whether the following measurements produce one triangle, two triangles or no triangle:
∠B = 88°, a = 23, b = 2. Solve if solution exists
If the sides of a ∆ABC are a = 4, b = 6 and c = 8, then show that 4 cos B + 3 cos C = 2
In a ∆ABC, if a = `sqrt(3) - 1`, b = `sqrt(3) + 1` and C = 60° find the other side and other two angles
In any ∆ABC, prove that the area ∆ = `("b"^2 + "c"^2 - "a"^2)/(4 cot "A")`
In a ∆ABC, if a = 12 cm, b = 8 cm and C = 30°, then show that its area is 24 sq.cm
In a ∆ABC, if a = 18 cm, b = 24 cm and c = 30 cm, then show that its area is 216 sq.cm
A straight tunnel is to be made through a mountain. A surveyor observes the two extremities A and B of the tunnel to be built from a point P in front of the mountain. If AP = 3 km, BP = 5 km, and ∠APB = 120°, then find the length of the tunnel to be built
A farmer wants to purchase a triangular-shaped land with sides 120 feet and 60 feet and the angle included between these two sides is 60°. If the land costs Rs.500 per square feet, find the amount he needed to purchase the land. Also, find the perimeter of the land
A plane is 1 km from one landmark and 2 km from another. From the plane’s point of view, the land between them subtends an angle of 45°. How far apart are the landmarks?
Two vehicles leave the same place P at the same time moving along two different roads. One vehicle moves at an average speed of 60 km/hr and the other vehicle moves at an average speed of 80 km/hr. After half an hour the vehicle reaches destinations A and B. If AB subtends 60° at the initial point P, then find AB
Suppose that a satellite in space, an earth station, and the centre of earth all lie in the same plane. Let r be the radius of earth and R he the distance from the centre of earth to the satellite. Let d be the distance from the earth station to the satellite. Let 30° be the angle of elevation from the earth station to the satellite, If the line segment connecting the earth station and satellite subtends angle α at the centre of earth then prove that d = `"R"sqrt(1 + ("r"/"R")^2 - 2 ("r"/"R") cos alpha)`
Choose the correct alternative:
In a triangle ABC, sin2A + sin2B + sin2C = 2, then the triangle is
