Question

A small bead of mass m can move on a smooth circular wire (radius R) under the action of a force F = `"Km"/"r"^2` directed (r = position of bead r from P and K = constant) towards a point P within the circle at a distance R/2 from the centre. The minimum velocity should be ______ m/s of bead at the point of the wire nearest the centre of force (P) so that bead will complete the circle. (Take `"k"/(3"R")` = 8 unit) 

Options

• 4

• 5

• 6

• 8

Answer 1

A small bead of mass m can move on a smooth circular wire (radius R) under the action of a force F = `"Km"/"r"^2` directed (r = position of bead r from P and K = constant) towards a point P within the circle at a distance R/2 from the centre. The minimum velocity should be 8 m/s of bead at the point of the wire nearest the centre of force (P) so that bead will complete the circle. (Take `"k"/(3"R")` = 8 unit) 

Explanation:

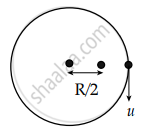
U = `-intvec"F".vec"dr"`

U = `-"km"/"r"`

K_i + U_i = K_f + U_f

`1/2"mv"^2-"km"/("R"/2) = 0 -"km"/((3"R")/2)`

`"mv"^2/2 = (2"Km")/"R"- (-2"Km")/(3"R")`

`"mv"^2/2 =(4"Km")/(3"R")`

V =`sqrt((8"K")/(3"R"))`, V = 8 m/s