Question

A solid sphere rolls down two different inclined planes of the same heights but different angles of inclination. (a) Will it reach the bottom with the same speed in each case? (b) Will it take longer to roll down one plane than the other? (c) If so, which one and why?

Answer 1

(a) Yes (b) Yes (c) On the smaller inclination

(a)Mass of the sphere = m

Height of the plane = h

Velocity of the sphere at the bottom of the plane = v

At the top of the plane, the total energy of the sphere = Potential energy = mgh

At the bottom of the plane, the sphere has both translational and rotational kinetic energies.

Hence, total energy = `1/2mv^2 + 1/2Iomega^2`

Using the law of conservation of energy, we can write:

`1/2mv^2 + 1/2 Iomega^2 = mgh`  ....(I)

For a solid sphere, the moment of inertia about its centre, `I = 2/3 mr^2`

Hence, equation (i) becomes:

`1/2 mv^2 +  1/2 (2/5 mr^2)omega^2 = mgh`

`1/2v^2 + 1/5r^2omega^2 =gh`

But we have the relation `v= r omega`

`:.1/2v^2 + 1/5v^2 = gh`

`v^2(7/10)  = gh`

`v = sqrt(10/7 gh)`

Hence, the velocity of the sphere at the bottom depends only on height (h) and acceleration due to gravity (g). Both these values are constants. Therefore, the velocity at the bottom remains the same from whichever inclined plane the sphere is rolled.

(b), (c)Consider two inclined planes with inclinations θ₁ and θ₂, related as: θ₁ < θ₂

The acceleration produced in the sphere when it rolls down the plane inclined at `theta_1` is  g sin `theta_1`

The various forces acting on the sphere are shown in the following figure.

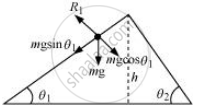
R₁ is the normal reaction to the sphere.

Similarly, the acceleration produced in the sphere when it rolls down the plane inclined at θ₂is:

g sin θ₂

The various forces acting on the sphere are shown in the following figure.

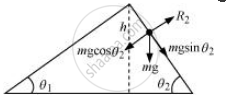
R₂ is the normal reaction to the sphere.

θ₂ > θ₁; sin θ₂ > sin θ₁ ... (i)

∴ a₂ > a_{1 …} (ii)

Initial velocity, u = 0

Final velocity, v = Constant

Using the first equation of motion, we can obtain the time of roll as:

v = u + at

`:. t prop 1/a`

`[("For inclination" theta_1: t_1 prop 1/a_1), ("For inclination" theta_2 : t_2 prop 1/a_2)]`   ...(iii)

From equations (ii) and (iii), we get:

t₂ < t₁

Hence, the sphere will take a longer time to reach the bottom of the inclined plane having the smaller inclination

Answer 2

(a) Using law of conservation of energy,

`1/2mv^2 + 1/2Iomega^2 = mgh`

or `1/2mv^2  + 1/2(2/5 mR^2) v^2/R^2 = mgh`

or 7/10v^2 = gh or v = `sqrt((10gh)/7)`

Since h is same for both the inclined planes therefore v is the same.

b) `l = 1/2((g sin theta)/(1+K^2/R^2)) t^2 = (g sin theta)/2(1+2/5) t^2 = (5g sin theta)/14 t^2`

or t = `sqrt((14l)/(5g sin theta)`

Now, `sin theta = h/l`  or l = `h/sin theta`

`:. t= 1/sin theta sqrt((14h)/"5g")`

Lesser the value of `theta` more will  be t

c) Clearly, the solid sphere will take longer to roll down the plane with smaller inclination.