Advertisements
Advertisements
Question
A triangular shaped glass with vertices at A(– 5, – 4), B(1, 6) and C(7, – 4) has to be painted. If one bucket of paint covers 6 square feet, how many buckets of paint will be required to paint the whole glass, if only one coat of paint is applied
Advertisements
Solution
Given the vertices of the triangular glass is A (– 5, – 4), B (1, 6), and C (7, – 4)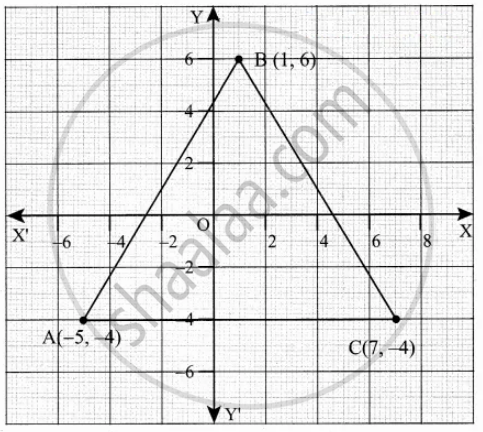
Area of triangle ACB = `1/2[(x_1y_2 + x_2y_3 + x_3y_1) - (x_2y_1 + x_3y_2 + x_1y_3)]`
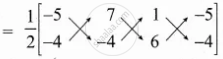
= `1/2[(20 + 42 - 4) - (-28 - 4 - 30)]`
= `1/2[58 - (- 62)]`
= `1/2 [58 + 62]`
= `1/2 xx 120`
= 60 sq. feet
Number of cans to paint 6 square feet = 1
∴ Number of cans = `60/6` = 10
⇒ Number of cans = 10
APPEARS IN
RELATED QUESTIONS
Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.
A hand fan is made by stitching lo equal size triangular strips of two different types of paper as shown in Fig. 12.28. The dimensions of equal strips are 25 cm, 25 cm and 14 cm. Find the area of each type of paper needed to make the hand fan.
Find the area of a triangle whose sides are 3 cm, 4 cm and 5 cm respectively.
Find the areas of the given plot. (All measures are in metres.)
Find the area of the triangle formed by the points
(–10, –4), (–8, –1) and (–3, –5)
The area of triangle formed by the points (− 5, 0), (0, – 5) and (5, 0) is
The perimeter of a triangular plot is 600 m. If the sides are in the ratio 5 : 12 : 13, then find the area of the plot
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 13 m, 14 m and 15 m. The advertisements yield an earning of Rs 2000 per m2 a year. A company hired one of its walls for 6 months. How much rent did it pay?
The perimeter of an isosceles triangle is 32 cm. The ratio of the equal side to its base is 3 : 2. Find the area of the triangle.
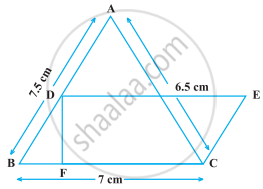
In the following figure, ∆ABC has sides AB = 7.5 cm, AC = 6.5 cm and BC = 7 cm. On base BC a parallelogram DBCE of same area as that of ∆ABC is constructed. Find the height DF of the parallelogram.
