Advertisements
Chapters
![Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board chapter 5 - Coordinate Geometry Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board chapter 5 - Coordinate Geometry - Shaalaa.com](/images/mathematics-english-class-10-sslc-tn-board_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 5: Coordinate Geometry
Below listed, you can find solutions for Chapter 5 of Tamil Nadu Board of Secondary Education Samacheer Kalvi for Mathematics [English] Class 10 SSLC TN Board.
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board 5 Coordinate Geometry Exercise 5.1 [Pages 211 - 212]
Find the area of the triangle formed by the points
(1, – 1), (– 4, 6) and (– 3, – 5)
Find the area of the triangle formed by the points
(–10, –4), (–8, –1) and (–3, –5)
Determine whether the sets of points are collinear?
`(-1/2, 3), (-5, 6) and (-8, 8)`
Determine whether the sets of points are collinear?
(a, b + c), (b, c + a) and (c, a + b)
Vertices of given triangles are taken in order and their areas are provided aside. Find the value of ‘p’.
| Vertices | Area (sq.units) |
| (0, 0), (p, 8), (6, 2) | 20 |
Vertices of given triangles are taken in order and their areas are provided aside. Find the value of ‘p’.
| Vertices | Area (sq.units) |
| (p, p), (5, 6), (5, –2) | 32 |
In the following, find the value of ‘a’ for which the given points are collinear
(2, 3), (4, a) and (6, – 3)
In the following, find the value of ‘a’ for which the given points are collinear
(a, 2 – 2a), (– a + 1, 2a) and (– 4 – a, 6 – 2a)
Find the area of the quadrilateral whose vertices are at (– 9, – 2), (– 8, – 4), (2, 2) and (1, – 3)
Find the area of the quadrilateral whose vertices are at (– 9, 0), (– 8, 6), (– 1, – 2) and (– 6, – 3)
Find the value of k, if the area of a quadrilateral is 28 sq. units, whose vertices are (– 4, – 2), (– 3, k), (3, – 2) and (2, 3)
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
Let P(11, 7), Q(13.5, 4) and R(9.5, 4) be the midpoints of the sides AB, BC and AC respectively of ∆ABC. Find the coordinates of the vertices A, B and C. Hence find the area of ∆ABC and compare this with area of ∆PQR.
The quadrilateral swimming pool shown is surrounded by concrete patio. Find the area of the patio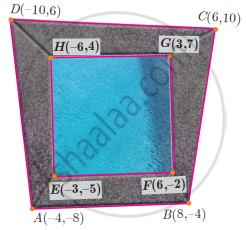
A triangular shaped glass with vertices at A(– 5, – 4), B(1, 6) and C(7, – 4) has to be painted. If one bucket of paint covers 6 square feet, how many buckets of paint will be required to paint the whole glass, if only one coat of paint is applied
Find the area of triangle AGF
Find the area of triangle FED
Find the area of quadrilateral BCEG
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board 5 Coordinate Geometry Exercise 5.2 [Pages 220 - 221]
What is the slope of a line whose inclination with positive direction of x-axis is 90°
What is the slope of a line whose inclination with positive direction of x-axis is 0°
What is the inclination of a line whose slope is 0
What is the inclination of a line whose slope is 1
Find the slope of a line joining the points
`(5, sqrt(5))` with the origin
Find the slope of a line joining the points
(sin θ, – cos θ) and (– sin θ, cos θ)
What is the slope of a line perpendicular to the line joining A(5, 1) and P where P is the mid-point of the segment joining (4, 2) and (–6, 4).
Show that the given points are collinear: (– 3, – 4), (7, 2) and (12, 5)
If the three points (3, – 1), (a, 3) and (1, – 3) are collinear, find the value of a
The line through the points (– 2, a) and (9, 3) has slope `-1/2` Find the value of a.
The line through the points (– 2, 6) and (4, 8) is perpendicular to the line through the points (8, 12) and (x, 24). Find the value of x.
Show that the given points form a right angled triangle and check whether they satisfy Pythagoras theorem
A(1, – 4), B(2, – 3) and C(4, – 7)
Show that the given points form a right angled triangle and check whether they satisfy Pythagoras theorem.
L(0, 5), M(9, 12) and N(3, 14)
Show that the given points form a parallelogram:
A(2.5, 3.5), B(10, – 4), C(2.5, – 2.5) and D(– 5, 5)
If the points A(2, 2), B(– 2, – 3), C(1, – 3) and D(x, y) form a parallelogram then find the value of x and y.
Let A(3, – 4), B(9, – 4), C(5, – 7) and D(7, – 7). Show that ABCD is a trapezium.
A quadrilateral has vertices at A(– 4, – 2), B(5, – 1), C(6, 5) and D(– 7, 6). Show that the mid-points of its sides form a parallelogram.
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board 5 Coordinate Geometry Exercise 5.3 [Pages 229 - 230]
Find the equation of a straight line passing through the mid-point of a line segment joining the points (1, – 5), (4, 2) and parallel to X-axis
Find the equation of a straight line passing through the mid-point of a line segment joining the points (1, – 5), (4, 2) and parallel to Y-axis
The equation of a straight line is 2(x – y) + 5 = 0. Find its slope, inclination and intercept on the Y-axis
Find the equation of a line whose inclination is 30˚ and making an intercept –3 on the Y-axis.
Find the slope and y intercept of `sqrt(3x) + (1 - sqrt(3))y` = 3
Find the value of ‘a’, if the line through (– 2, 3) and (8, 5) is perpendicular to y = ax + 2
The hill in the form of a right triangle has its foot at (19, 3). The inclination of the hill to the ground is 45°. Find the equation of the hill joining the foot and top.
Find the equation of a line through the given pair of point `(2,2/3)` and `((-1)/2, -2)`
Find the equation of a line through the given pair of point (2, 3) and (– 7, – 1)
A cat is located at the point(– 6, – 4) in xy plane. A bottle of milk is kept at (5, 11). The cat wish to consume the milk travelling through shortest possible distance. Find the equation of the path it needs to take its milk.
Find the equation of the median and altitude of ΔABC through A where the vertices are A(6, 2), B(– 5, – 1) and C(1, 9)
Find the equation of a straight line which has slope `(−5)/4` and passing through the point (– 1, 2).
You are downloading a song. The percent y (in decimal form) of megabytes remaining to get downloaded in x seconds is given by y = – 0.1x + 1 find the total MB of the song.
You are downloading a song. The percent y (in decimal form) of megabytes remaining to get downloaded in x seconds is given by y = – 0.1x + 1 after how many seconds will 75% of the song gets downloaded?
You are downloading a song. The percent y (in decimal form) of megabytes remaining to get downloaded in x seconds is given by y = – 0.1x + 1 after how many seconds the song will be downloaded completely?
Find the equation of a line whose intercepts on the x and y axes are given below
4, – 6
Find the equation of a line whose intercepts on the x and y axes are given below
`- 5, 3/4`
Find the intercepts made by the following line on the coordinate axes
3x – 2y – 6 = 0
Find the intercepts made by the following line on the coordinate axes
4x + 3y + 12 = 0
Find the equation of a straight line passing through (1, – 4) and has intercepts which are in the ratio 2 : 5
Find the equation of a straight line passing through (– 8, 4) and making equal intercepts on the coordinate axes
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board 5 Coordinate Geometry Exercise 5.4 [Page 235]
Find the slope of the following straight line
5y – 3 = 0
Find the slope of the following straight line
`7x - 3/17` = 0
Find the slope of the line which is parallel to y = 0.7x – 11
Find the slope of the line which is perpendicular to the line x = – 11
Check whether the given lines are parallel or perpendicular
`x/3 + y/4 + 1/7` = 0 and `(2x)/3 + y/2 + 1/10` = 0
Check whether the given lines are parallel or perpendicular
5x + 23y + 14 = 0 and 23x – 5x + 9 = 0
If the straight lines 12y = − (p + 3)x + 12, 12x – 7y = 16 are perpendicular then find ‘p’
Find the equation of a straight line passing through the point P(−5, 2) and parallel to the line joining the points Q(3, −2) and R(−5, 4)
Find the equation of a line passing through (6, −2) and perpendicular to the line joining the points (6, 7) and (2, −3)
A(−3, 0) B(10, −2) and C(12, 3) are the vertices of ∆ABC. Find the equation of the altitude through A and B.
Find the equation of the perpendicular bisector of the line joining the points A(− 4, 2) and B(6, − 4)
Find the equation of a straight line through the intersection of lines 7x + 3y = 10, 5x – 4y = 1 and parallel to the line 13x + 5y + 12 = 0
Find the equation of a straight line through the intersection of lines 5x – 6y = 2, 3x + 2y = 10 and perpendicular to the line 4x – 7y + 13 = 0
Find the equation of a straight line joining the point of intersection of 3x + y + 2 = 0 and x – 2y – 4 = 0 to the point of intersection of 7x – 3y = – 12 and 2y = x + 3
Find the equation of a straight line through the point of intersection of the lines 8x + 3y = 18, 4x + 5y = 9 and bisecting the line segment joining the points (5, −4) and (−7, 6)
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board 5 Coordinate Geometry Exercise 5.5 [Pages 235 - 236]
Multiple choice questions
The area of triangle formed by the points (− 5, 0), (0, – 5) and (5, 0) is
0 sq.units
25 sq.units
5 sq.units
none of these
A man walks near a wall, such that the distance between him and the wall is 10 units. Consider the wall to be the Y-axis. The path travelled by the man is
x = 10
y = 10
x = 0
y = 0
The straight line given by the equation x = 11 is
parallel to X-axis
parallel to Y-axis
passing through the origin
passing through the point (0, 11)
If (5, 7), (3, p) and (6, 6) are collinear, then the value of p is
3
6
9
12
The point of intersection of 3x – y = 4 and x + 7 = 8 is
(5, 3)
(2, 4)
(3, 5)
(4, 4)
The slope of the line joining (12, 3), (4, a) is `1/8`. The value of ‘a’ is
1
4
− 5
2
The slope of the line which is perpendicular to a line joining the points (0, 0) and (− 8, 8) is
− 1
1
`1/3`
− 8
If slope of the line PQ is `1/sqrt(3)` then slope of the perpendicular bisector of PQ is
`sqrt(3)`
`-sqrt(3)`
`1/sqrt(3)`
0
If A is a point on the Y-axis whose ordinate is 8 and B is a point on the X-axis whose abscissae is 5 then the equation of the line AB is
8x + 5y = 40
8x – 5y = 40
x = 8
y = 5
The equation of a line passing through the origin and perpendicular to the line 7x − 3y + 4 = 0 is
7x – 3y + 4 = 0
3x – 7y + 4 = 0
3x + 7y = 0
7x – 3y = 0
Consider four straight lines
(i) l1; 3y = 4x + 5
(ii) l2; 4y = 3x – 1
(iii) l3; 4y + 3x = 7
(iv) l4; 4x + 3y = 2
Which of the following statement is true?
l1 and l2 are perpendicular
l2 and l4 are parallel
l2 and l4 are perpendicular
l2 and l3 are parallel
A straight line has equation 8y = 4x + 21. Which of the following is true
The slope is 0.5 and the y intercept is 2.6
The slope is 5 and the y intercept is 1.6
The slope is 0.5 and the y intercept is 1.6
The slope is 5 and the y intercept is 2.6
When proving that a quadrilateral is a trapezium, it is necessary to show
Two sides are parallel
Two parallel and two non-parallel sides
Opposite sides are parallel
All sides are of equal length
When proving that a quadrilateral is a parallelogram by using slopes you must find
The slopes of two sides
The slopes of two pair of opposite sides
The lengths of all sides
Both the lengths and slopes of two sides
(2, 1) is the point of intersection of two lines
x – y – 3 = 0; 3x – y – 7 = 0
x + y = 3; 3x + y = 7
3x + y = 3; x + y = 7
x + 3y – 3 = 0; x – y – 7 = 0
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board 5 Coordinate Geometry Unit Exercise – 5 [Page 237]
PQRS is a rectangle formed by joining the points P(– 1, – 1), Q(– 1, 4), R(5, 4) and S(5, – 1). A, B, C and D are the mid-points of PQ, QR, RS and SP respectively. Is the quadrilateral ABCD a square, a rectangle or a rhombus? Justify your answer.
The area of a triangle is 5 sq. units. Two of its vertices are (2, 1) and (3, −2). The third vertex is (x, y) where y = x + 3. Find the coordinates of the third vertex.
Find the area of a triangle formed by the lines 3x + y – 2 = 0, 5x + 2y – 3 = 0 and 2x – y – 3 = 0
If vertices of a quadrilateral are at A(– 5, 7), B(– 4, k), C(– 1, – 6) and D(4, 5) and its area is 72 sq. units. Find the value of k.
Without using distance formula, show that the points (−2, −1), (4, 0), (3, 3) and (−3, 2) are vertices of a parallelogram
Find the equations of the lines, whose sum and product of intercepts are 1 and – 6 respectively
The owner of a milk store finds that he can sell 980 litres of milk each week at ₹ 14/litre and 1220 litres of milk each week at ₹ 16/litre. Assuming a linear relationship between selling price and demand, how many litres could he sell weekly at ₹ 17/litre?
Find the image of the point (3, 8) with respect to the line x + 3y = 7 assuming the line to be a plane mirror.
Find the equation of a line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes.
A person standing at a junction (crossing) of two straight paths represented by the equations 2x – 3y + 4 = 0 and 3x + 4y – 5 = 0 seek to reach the path whose equation is 6x – 7y + 8 = 0 in the least time. Find the equation of the path that he should follow.
Solutions for 5: Coordinate Geometry
![Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board chapter 5 - Coordinate Geometry Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board chapter 5 - Coordinate Geometry - Shaalaa.com](/images/mathematics-english-class-10-sslc-tn-board_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Samacheer Kalvi solutions for Mathematics [English] Class 10 SSLC TN Board chapter 5 - Coordinate Geometry
Shaalaa.com has the Tamil Nadu Board of Secondary Education Mathematics Mathematics [English] Class 10 SSLC TN Board Tamil Nadu Board of Secondary Education solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Samacheer Kalvi solutions for Mathematics Mathematics [English] Class 10 SSLC TN Board Tamil Nadu Board of Secondary Education 5 (Coordinate Geometry) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Samacheer Kalvi textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 10 SSLC TN Board chapter 5 Coordinate Geometry are Area of a Triangle by Heron's Formula, Inclination of a Line, Straight Line, General Form of a Straight Line, Coordinate Geometry, Area of a General Quadrilateral.
Using Samacheer Kalvi Mathematics [English] Class 10 SSLC TN Board solutions Coordinate Geometry exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Samacheer Kalvi Solutions are essential questions that can be asked in the final exam. Maximum Tamil Nadu Board of Secondary Education Mathematics [English] Class 10 SSLC TN Board students prefer Samacheer Kalvi Textbook Solutions to score more in exams.
Get the free view of Chapter 5, Coordinate Geometry Mathematics [English] Class 10 SSLC TN Board additional questions for Mathematics Mathematics [English] Class 10 SSLC TN Board Tamil Nadu Board of Secondary Education, and you can use Shaalaa.com to keep it handy for your exam preparation.
