Advertisements
Advertisements
Question
A uniform metre rule is pivoted at its mid-point. A weight of 50 gf is suspended at one end of it. Where should a weight of 100 gf be suspended to keep the rule horizontal?
Solution
Length of meter scale = 1 m
Weight W1 = 50 gf
Weight W2 = 100 gf
distance = ?
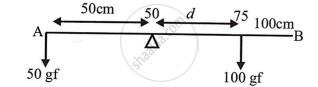
W1 × d = W2 × d
50 × 50 cm = 100 × d
d = `(50 ×50)/100 = 25` cm from other end
APPEARS IN
RELATED QUESTIONS
Describe a simple experiment to verify the principle of moments, if you are supplied with a metre rule, a fulcrum and two springs with slotted weights.
The diagram below shows a uniform bar supported at the middle point O. A weight of 40 gf is placed at a distance of 40 cm to the left of the point O. How can you balance the bar with a weight of 80 gf?

When a boy weighing 20 kgf sits at one end of a 4 m long see-saw, it gets depressed at this end. How can it be brought to the horizontal position by a man weighing 40 kgf.
A physical balance has its arms of length 60 cm and 40 cm. What weight kept on pan of the longer arm will balance an object of weight 100 gf kept on other pan?
A uniform metre rule of mass 100 g is balanced on the fulcrum at mark 40 cm by suspending an unknown mass m at the mark 20 cm.
- Find the value of m.
- To which side the rule will tilt if the mass m is moved to the mark 10 cm?
- What is the resultant moment now?
- How can it be balanced by another mass of 50 g?
A uniform metre rule balances horizontally on a knife edge placed at the 58 cm mark when a weight of 20 gf is suspended from one end.
- Draw a diagram of the arrangement.
- What is the weight of the rule?
A uniform metre rule of weight 10 gf is pivoted at its 0 mark.
- What moment of force depresses the rule?
- How can it be made horizontal by applying a least force?
A uniform half-metre rule can be balanced at the 29.0 cm mark when a mass of 20 g is hung from its one end.
- Draw a diagram of the arrangement.
- Find the mass of the half-metre rule.
- In which direction would the balancing point shift if 20 g mass is shifted inside from its one end?
A uniform metre rule of mass 100g is balanced on a fulcrum at mark 40cm by suspending an unknown mass m at the mark 20cm. What is the resultant moment now?
A uniform metre rule of mass 100g is balanced on a fulcrum at mark 40cm by suspending an unknown mass m at the mark 20cm.
How can it be balanced by another mass 50 g ?
