Advertisements
Advertisements
Question
ABCD is a parallelogram in which AE is perpendicular to CD as shown in the given figure. Also, AC = 5 cm, DE = 4 cm and area of ΔAED = 6 cm2. Find the perimeter and area of parallelogram ABCD.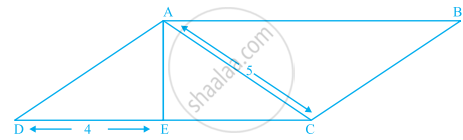
Solution
Given area of ΔAED = 6 cm2 and AC = 5 cm and DE = 4 cm
∴ Area of ΔAED = `1/2` × DE × AE ......[∵ Area of triangle = Base × Height]
⇒ `1/2` × 4 × AE = 6
⇒ AE = `(6 xx 2)/4`
⇒ AE = 3 cm
Now, In right-angled ΔAEC, AE = 3 cm and AC = 5 cm
So, = (EC)2 = (AC)2 – (AE)2 ......[By Pythagoras theorem]
⇒ (EC)2 = 52 – 32 = 25 – 9
⇒ EC = `sqrt(16)`
⇒ EC = 4 cm
∵ DE + EC = DC
⇒ DC = 4 + 4 = 8 cm
∵ ABCD is a parallelogram
So, AB = DC = 8 cm
Now, In right-angled ΔAED, AD2 = AE2 + ED2 ......[By Pythagoras theorem]
⇒ AD2 = 32 + 42 = 9 + 16
⇒ AD = `sqrt(25)`
⇒ AD = 5 cm
So, AD = BC = 5 cm ......[∵ ABCD is a parallelogram]
∴ Perimeter of paralielogram ABCD = 2(l + b) = 2(DC + AD) = 2(8 + 5) = 2 × 13 = 26 cm
Area of parallelogram ABCD = Base × Height = DC × AE = 8 × 3 = 24 cm.
APPEARS IN
RELATED QUESTIONS
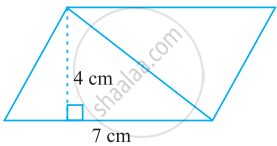
Find the area of the following parallelogram:
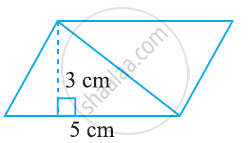
Find the area of the following parallelogram:
If base of a parallelogram is 18 cm and its height is 11 cm, find its area.
Area of a parallelogram is 83.2 sq cm. If its height is 6.4 cm, find the length of its base.
Find the height ‘x’ if the area of the parallelogram is 24 cm2 and the base is 4 cm.
The area of parallelogram whose base 10 m and height 7 m is
The base of the parallelogram with area is 52 sq.cm and height 4 cm is
The height of the parallelogram is one-fourth of its base. If the area of the parallelogram is 676 sq.cm, find the height and the base
The area of the parallelogram ABCD is 1470 sq.cm. If AB = 49 cm and AD = 35 cm then, find the height, DF and BE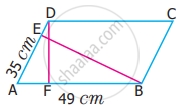
Area of parallelogram ABCD (see the figure) is not equal to ______.