Advertisements
Advertisements
Question
Add the following rational numbers:
Solution
\[\text{The L.C.M. of denominators 16 and 24 is 48} . \]
\[\text{Now, we will express} \frac{- 5}{16} \text{and} \frac{7}{24} \text{in the form in which they take the denominator 48} . \]
\[\frac{- 5 \times 3}{16 \times 3} = \frac{- 15}{48} \]
\[\frac{7 \times 2}{24 \times 2} = \frac{14}{48}\]
\[\text{So}\]
\[\frac{- 5}{16} + \frac{7}{24} = \frac{- 15}{48} + \frac{14}{48}\]
\[ = \frac{( - 15) + 14}{48}\]
\[ = \frac{- 15 + 14}{48}\]
\[ = \frac{- 1}{48}\]
APPEARS IN
RELATED QUESTIONS
Add and express the sum as a mixed fraction:
Re-arrange suitably and find the sum in each of the following:
Evaluate each of the following:
Fill in the branks:
Simplify each of the following and express the result as a rational number in standard form:
Simplify:
Fill in the blanks:
The numbers ..... and ..... are their own reciprocals.
The numerical expression `3/8 + ((-5))/7 = (-19)/56` shows that ______
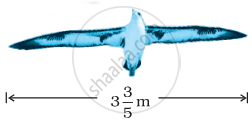
The diagram shows the wingspans of different species of birds. Use the diagram to answer the question given below:
| Albatross | Sea Gull |
 |
 |
How much longer is the wingspan of an Albatross than the wingspan of a Sea gull?
Write the following numbers in the form `p/q`, where p and q are integers:
One-fourth
