Question

Answer in brief:

Derive an expression which relates angular momentum with the angular velocity of a rigid body​.

Answer 1

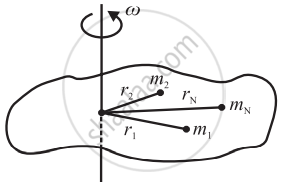
The figure above shows a rigid object rotating with a constant angular speed ω about an axis perpendicular to the plane of paper. For theoretical simplification let us consider the object to be consisting of N number of particles of masses m₁, m₂, ....., m_N at respective perpendicular distances r₁, r₂,..., r_N, respectively from the axis of rotation. As the object rotates, all these particles perform UCM with same angular speed ω , but with different linear speeds `"v"_1=r_1omega`, `"v"_2=r_2omega`, ..., `"v"_N=r_Nomega`.

Directions of individual velocities `vec"v"_1`, `vec"v"_2`, ..., `vec"v"_N` are along the tangents to their respective tracks.

Linear momentum of the first particle is of magnitude

`p_1 = m_1"v"_1= "m"_1"r"_1omega`

Its angular momentum, defined by `vec"L"_1 = vec"p"_1 xx vec"r"_1`, is thus of magnitude

`"L"_1 = "p"_1"r"_1 = "m"_1"r"_1omega"r"_1 = "m"_1"r"_1^2omega`

Similarly, `"L"_2 = "m"_2"r"_2^2omega`, `"L"_3 = "m"_3"r"_3^2omega`, ..., `"L"_N = "m"_N"r"_N^2omega`

The angular momentum of the body about the given axis is

L = `"L"_1 + "L"_2 + ... +"L"_"N"`

`= "m"_1"r"_1^2omega + "m"_2"r"_2^2omega + ...+ "m"_"N""r"_"N"^2 omega`

`= ("m"_1"r"_1^2 + "m"_2"r"_2^2 +... + "m"_"N""r"_"N"^2)omega`

∴ L = Iω

where I = `("m"_1"r"_1^2 + "m"_2"r"_2^2 +... + "m"_"N""r"_"N"^2)` is the moment of inertia of the body about the given axis of rotation.

In vector form, `vec"L" = "I"vecomega`

Thus, angular momentum = moment of inertia × angular velocity.