Advertisements
Advertisements
Question
Answer the following question.
In a series LCR circuit connected across an ac source of variable frequency, obtain the expression for its impedance and draw a plot showing its variation with frequency of the ac source.
Solution
Consider the LCR circuit
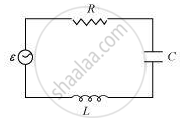
An AC source E with voltage `ν = ν_m` sin ωt is applied across LCR circuit

As the inductor, capacitor, and the resistor are connected in series so the current through all of them is the same (same amplitude and same phase)
Let the current be 1= Im sin wt
The voltage across each component has a different phase relation with the current.
- Let the maximum voltage across the resistor be VR = ImR that is in the same phase of the current hence it is represented by OA in the phasor diagram.
- Let the maximum voltage across the inductor be VL = ImXL and that leads the current by `pi/2` it is represented by OD in the phasor diagram.
- Let the maximum voltage across the capacitor be Vc = ImXc and that lags behind the current by `pi/2`, it is represented by OC in the phasor diagram.
Resultant voltage can be found by using the vector sum of the phasors. The resultant voltage is represented by OF.
It can be written as:
`V_m = sqrt(V_R^2 + (V_L - V_c)^2)`
`V_m = sqrt((I_mR)^2 + (I_mX_L - I_mX_c)^2)`
`V_m = I_m sqrt(R^2 + (X_L - X_c)^2)`
`Z = V_m/I_m = sqrt(R^2 + (X_L - X_c)^2)`
or, `Z = V_m/I_m = sqrt(R^2 + (ωL - 1/(ωC))^2)`
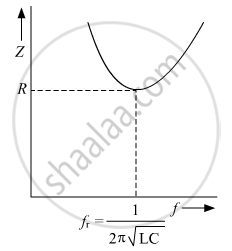
Variation of impedance Z with frequency f:
RELATED QUESTIONS
Define 'quality factor' of resonance in a series LCR circuit. What is its SI unit?
Why does current in a steady state not flow in a capacitor connected across a battery? However momentary current does flow during charging or discharging of the capacitor. Explain.
Show that in an a.c. circuit containing a pure inductor, the voltage is ahead of current by π/2 in phase ?
A coil having an inductance L and a resistance R is connected to a battery of emf ε. Find the time taken for the magnetic energy stored in the circuit to change from one fourth of the steady-state value to half of the steady-state value.
Derive an expression for the average power dissipated in a series LCR circuit.
A series LCR circuit with R = 20 Ω, L = 1.5 H and C = 35 µF is connected to a variable-frequency 200 V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit in one complete cycle?
In an LCR circuit having L = 8 henery. C = 0.5 µF and R = 100 ohm in series, the resonance frequency in radian/sec is
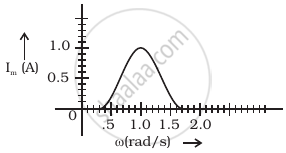
In series LCR circuit, the plot of Imax vs ω is shown in figure. Find the bandwidth and mark in the figure.
A series LCR circuit driven by 300 V at a frequency of 50 Hz contains a resistance R = 3 kΩ, an inductor of inductive reactance XL = 250 πΩ, and an unknown capacitor. The value of capacitance to maximize the average power should be ______.
Three students, X, Y and Z performed an experiment for studying the variation of a.c. with frequency in a series LCR circuit and obtained the graphs as shown below. They all used
- an AC source of the same emf and
- inductance of the same value.
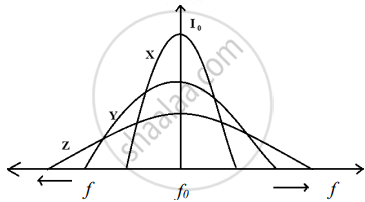
- Who used minimum resistance?
- In which case will the quality Q factor be maximum?
- What did the students conclude about the nature of impedance at resonant frequency (f0)?
- An ideal capacitor is connected across 220V, 50Hz, and 220V, 100Hz supplies. Find the ratio of current flowing through it in the two cases.
