Advertisements
Advertisements
Question
Calculate the equivalent resistance of the following combination of resistor r1, r2, r3, and r4
Advertisements
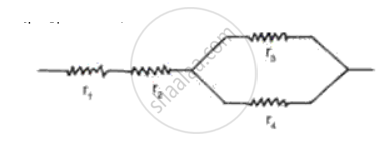
Solution 1
In the given network, the series combination of resistors, r1 and r2 is connected in series with the parallel combination of resistors, r3 and r4
Equivalent resistance of resistor r1 and r2 , Rs= r1 + r2
Equivalent resistance of resistor r3 and r4 Rp = `[1/ "r"^3 + 1/"r"^4]^-1 = ("r"_3"r"_4)/("r"_3 + "r"_4)`
equivalent resistance of the given network, R = Rs + Rp = r1 + r2 + `("r"_3"r"_4)/("r"_3 + "r"_4)`
Solution 2
Since r3 and r4 are in parallel
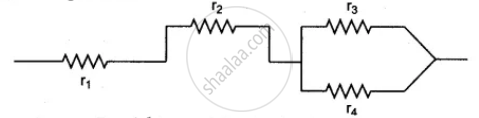
∴ Equivalent resistance R1 of this combination is given by
`1/"R"_1 = 1/"r"_3 + 1/"r"_4 = ("r"_4 + "r"_3)/("r"_4 "r"_3)`
or R1 = `("r"_3 "r"_4)/("r"_3 + "r"_4)`
Now r1, r2 and R1 are in series
∴ Equivalent resistance R of the whole combination is
R = r1 + r2 + `("r"_3 "r"_4)/("r"_3 + "r"_4)`
= `(("r"_1 + "r"_2)("r"_3 + "r"_4) + "r"_3"r"_4)/("r"_3 + "r"_4) Omega`
APPEARS IN
RELATED QUESTIONS
Give one example to show how the resistance depends on the nature of material of the conductor.
Give the law of combination of resistances in series.
How will you oonnect five resistors, each of the value one ohm, to obtain an equivalent resistance of 0.2 `Omega`
What do you mean by power rating of an electrical appliance? How do you use it to calculate
(a) the resistance of the appliance and
(b) the safe limit of the current in it, while in use?
A given metallic wire of resistance R is doubled on itself. What will be its new resistance?
State the effect of rise in temperature on the resistance of semi-conductors.
How does the resistivity of an alloy such as constantace depends on temperature.
Illustrate-combination of cells e.g., three cells, in series, explaining the combination briefly. Obtain an expression for current ‘i’ in the combination.
Two wires of same material and same lengths have radii in the ratio of 2 : 3, Compare their resistances.
