Advertisements
Advertisements
Question
Consider two identical point charges located at points (0, 0) and (a, 0).
Is there a point on the line joining them at which the electric field is zero?
Solution
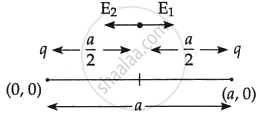
Let at `a/2`:
`E_1 = (Kq)/(a"/"2)^2`
`E_2 = (Kq)/(a"/"2)^2`
The net electric field at a/2:
Enet = E1 - E2
∵ E1 = E2
Enet = 0
at `a/2`
So `a/2` is a point where the electric field is zero.
APPEARS IN
RELATED QUESTIONS
What is the amount of work done in moving a point charge Q around a circular arc of radius ‘r’ at the centre of which another point charge ‘q’ is located?
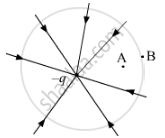
The field lines of a negative point charge are as shown in the figure. Does the kinetic energy of a small negative charge increase or decrease in going from B to A?
What is the function of uniform radial field and how is it produced?
Why must electrostatic field at the surface of a charged conductor be normal to the surface at every point? Give reason?
A charged oil drop weighing 1.6 x 10-15 N is found to remain suspended in a uniform electric field of intensity 2 x 103 Nc-1. Find the charge on the drop.
A solid sphere of radius R has a charge Q distributed in its volume with a charge density ρ = kra, where k and a are constants and r is the distance from its centre. If the electric field at r = `"R"/2` is `1/8` times that at r = R, the value of a is ______.
A particle of charge q and mass m moves rectilinearly under the action of electric field E = A – Bx, where A and B are positive constants and x is distance from the point where particle was initially at rest then the distance traveled by the particle before coming to rest and acceleration of particle at that moment are respectively.
Let there be a spherically symmetric charge distribution with charge density varying as `rho("r") = rho_0(5/4 - "r"/"R")` upto r = R, and `rho("r") = 0` for r > R, where r is the distance from the origin. The electric field at a distance r(r < R) from the origin is given by _________.
