Advertisements
Advertisements
Question
Determine the tension in a cable BC shown in fig by virtual work method.

Given: F=3500 N
ϴ = 50o
Length of rod = 3.75 mm + 1.5 mm = 5.25 mm
To find : Tension in cable BC
Solution
Let rod AB have a small virtual angular displacement θ in the clockwise direction
No virtual work will be done by the reaction force RA since it is not an active force
Assuming weight of rod to be negligible
Let A be the origin and dotted line through A be the X-axis of the system
| Active force(N) | Co-ordinate of the point of action along the force | Virtual displacement |
| 3500 | Y co-ordinate of D=yD=3.75sin θ | 𝛿yD=3.75cos θ𝛿θ |
| Tcos30 | X co-ordinate of B=xB=5.25cos θ | 𝛿xB=-5.25sin θ𝛿θ |
| Tsin30 | Y co-ordinate of B=yB=5.25sin θ | 𝛿yB=5.25cos θ𝛿θ |
By principle of virtual work :
-3500 x yd -Tsin 30 x yB -Tcos 30 x xB =0
-3500(3.75cos θ𝛿θ) – Tsin30(5.25cos θ𝛿θ) -Tcos30(-5.25sin θ 𝛿θ) = 0
Putting value of θ = 50o and dividing the above equation by 𝛿θ
(-3500 x 3.75cos50) – (Tsin30 x 5.25 cos50) + (Tcos30 x 5.25 sin50) = 0
5.25T(-sin30.cos50 + cos30.sin 50) = 3500 x 3.75 cos 50
`T=(3500∗3.75c0s50)/(5.25(cos 30.sin50-sin30.cos50)`
`=(3500∗3.75cos50)/(5.25sin20)`
`=4698.4631 N`
The tension in the cable BC is 4698.4631 N
APPEARS IN
RELATED QUESTIONS
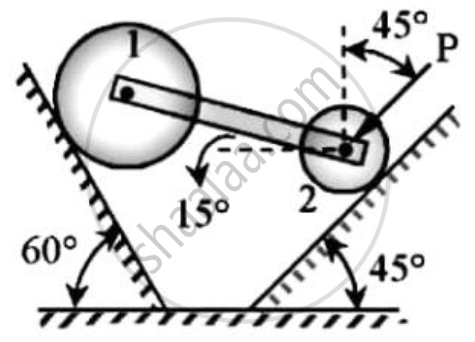
Two cylinders 1 and 2 are connected by a rigid bar of negligible weight hinged to each cylinder and left to rest in equilibrium in the position shown under the application of force P applied at the center of cylinder 2.
Determine the magnitude of force P.If the weights of the cylinders 1 and 2 are 100N and 50 N respectively.
Determine the required stiffness k so that the uniform 7 kg bar AC is in equilibrium when θ=30o.
Due to the collar guide at B the spring remains vertical and is unstretched when θ = 0o.Use principle of virtual work.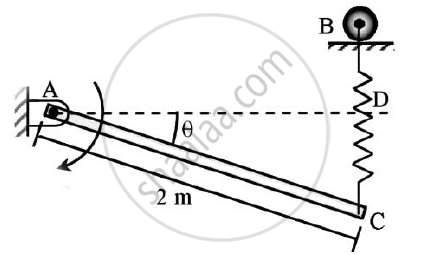
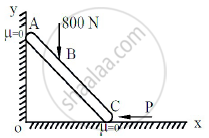
Using Principle of Virtual Work, determine the force P which will keep the weightless bar AB in equilibrium. Take length AB as 2m and length AC as 8m. The bar makes an angle of 30° with horizontal. All the surfaces in contact are smooth. Refer Figure 9.