Advertisements
Advertisements
Question
Find the acceleration due to gravity of the moon at a point 1000 km above the moon's surface. The mass of the moon is 7.4 × 1022 kg and its radius is 1740 km.
Solution
The acceleration due to gravity at a point at height h from the surface of the moon is given by \[g = \frac{GM}{r^2}\] ,
where M is the mass of the moon; r is the distance of point from the centre of the moon and G is universal gravitational constant.
\[\therefore g = \frac{GM}{\left( R + h \right)^2}\]
\[ \Rightarrow g = \frac{6 . 67 \times {10}^{- 11} \times 7 . 4 \times {10}^{22}}{\left( 1740 + 1000 \right)^2 \times {10}^6}\]
\[ \Rightarrow g = \frac{6 . 67 \times 7 . 4 \times {10}^{11}}{\left( 1740 + {1000}^2\times {10}^6 \right)}\]
\[ \Rightarrow g = \frac{6 . 67 \times 7 . 4 \times {10}^{11}}{2740 \times 2740 \times {10}^6}\]
\[ \Rightarrow g = 0 . 65 m/ s^2\]
APPEARS IN
RELATED QUESTIONS
Is there any meaning of "Weight of the earth"?
If heavier bodies are attracted more strongly by the earth, why don't they fall faster than the lighter bodies?
The acceleration of moon with respect to earth is 0⋅0027 m s−2 and the acceleration of an apple falling on earth' surface is about 10 m s−2. Assume that the radius of the moon is one fourth of the earth's radius. If the moon is stopped for an instant and then released, it will fall towards the earth. The initial acceleration of the moon towards the earth will be
The acceleration of the moon just before it strikes the earth in the previous question is
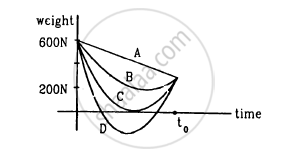
Suppose, the acceleration due to gravity at the earth's surface is 10 m s−2 and at the surface of Mars it is 4⋅0 m s−2. A 60 kg passenger goes from the earth to the Mars in a spaceship moving with a constant velocity. Neglect all other objects in the sky. Which part of the following figure best represents the weight (net gravitational force) of the passenger as a function of time?
Find the acceleration due to gravity in a mine of depth 640 m if the value at the surface is 9.800 m s−2. The radius of the earth is 6400 km.
A particle is fired vertically upward with a speed of 15 km s−1. With what speed will it move in interstellar space. Assume only earth's gravitational field.
Calculate the change in g value in your district of Tamil nadu. (Hint: Get the latitude of your district of Tamil nadu from Google). What is the difference in g values at Chennai and Kanyakumari?
One can easily weigh the earth by calculating the mass of the earth by using the formula:
Which of the following options are correct?
- Acceleration due to gravity decreases with increasing altitude.
- Acceleration due to gravity increases with increasing depth (assume the earth to be a sphere of uniform density).
- Acceleration due to gravity increases with increasing latitude.
- Acceleration due to gravity is independent of the mass of the earth.
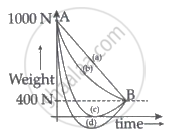
A person whose mass is 100 kg travels from Earth to Mars in a spaceship. Neglect all other objects in the sky and take acceleration due to gravity on the surface of the Earth and Mars as 10 m/s2 and 4 m/s2 respectively. Identify from the below figures, the curve that fits best for the weight of the passenger as a function of time.
If R is the radius of the earth and g is the acceleration due to gravity on the earth's surface, the mean density of the earth is ______.
A ball is immersed in water kept in container and released. At the same time container is accelerated in horizontal direction with acceleration, `sqrt44` m/s2. Acceleration of ball w.r.t. container is ______ m/s2 (specific gravity of ball = 12/17, g = 10 m/s2)
If the radius of the earth shrinks by 2% while its mass remains the same. The acceleration due to gravity on the earth's surface will approximately ______.
The percentage decrease in the weight of a rocket, when taken to a height of 32 km above the surface of the earth will, be ______.
(Radius of earth = 6400 km)
