Advertisements
Advertisements
Question
Draw an angle of 110° with the help of a protractor and bisect it. Measure each angle.
Solution
Draw ∠BXA = 110° with the help of a protractor.
Now, we use the following steps for required construction
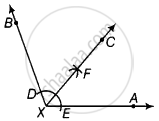
1. Taking X as centre and any radius draw an arc to intersect the rays XA and XB, say at E and D, respectively.
2. Taking D and E as centres and with the radius more than `1/2` DE, draw arcs to intersect each other, say at F.
3. Draw the ray XF. Thus, ray XF is the required bisector of the angle BXA. On measuring each angle, we get
∠BXC = ∠AXC = 55° ...[∴ ∠BXC = ∠AXC = `1/2` ∠BXA = `1/2` × 110° = 55°]
APPEARS IN
RELATED QUESTIONS
Construct an angle of 45° at the initial point of a given ray and justify the construction.
Construct the 135° angles and verify by measuring it by a protractor.
Draw an angle and label it as ∠BAC. Construct another angle, equal to ∠BAC.
Draw an obtuse angle, Bisect it. Measure each of the angles so obtained.
Using your protractor, draw an angle of measure 108°. With this angle as given, draw an
angle of 54°.
Draw a linear pair of angles. Bisect each of the two angles. Verify that the two bisecting rays are perpendicular to each other.
Using ruler and compasses only, draw an angle of measure 135°.
Construct the angle of the measurement:
1. 135°
Construct the angle of the measurement:
1. 15°
Construct the angle of the measurement:
1. 22 `(1°)/2`
