Question

Draw a triangle ABC with BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are`4/5` times the corresponding sides of ΔABC.

Answer 1

Given:
∠B = 45°
∠A = 105°

Sum of all interior angles in a triangle is 180°.
∠A + ∠B + ∠C = 180°

105° + 45° + ∠C = 180°

∠C = 180° − 150°

∠C = 30°

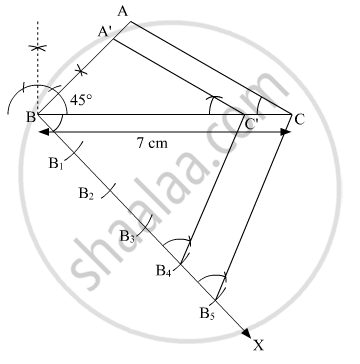
Following steps are involved in the construction of the required triangle:

Step 1

Draw a ΔABC with side BC = 7 cm, ∠B = 45° and ∠C = 30°.

Step 2

Draw a ray BX making an acute angle with BC on the opposite side of vertex A.

Step 3

Locate 5 points (as 5 is greater in 5 and 4) B_1, B_2, B_3, B₄ and B₅ on BX such that BB₁, B₁B₂, B₂B₃, B₃B₄ and B₄B₅.

Step 4

Join B₅C. Draw a line through B₄ parallel to B₅C intersecting extended BC at C'.

Step 5

Through C', draw a line parallel to AC intersecting BA at A'.

ΔA'BC' is the required triangle.

Justification

The construction can be justified by proving that

 `A'B=4/5AB,BC'=4/5BC andA'C,= 4/5AC`

 In ΔABC and ΔA'BC',

∠ABC = ∠A'BC' (Common)

∠ACB = ∠A'C'B (Corresponding angles)

∴ ΔABC ∼ ΔA'BC' (AA similarity criterion)

`(AB)/(A'B)=(BC)/(BC')=(AC)/(A'C')`

In ΔBB₅C and ΔBB₄C',

∠B₄BC' = ∠B₅BC (Common)

∠BB₄C' = ∠BB₅C' (Corresponding angles)

∴ ΔBB₄C' ∼ ΔBB₅C (AA similarity criterion)

`(BC')/(BC)=(BB_4)/(BB_6)`

`=>(BC')/(BC)=4/5 `

Comparing (1) and (2), we obtain

 `(A'B)/(AB)=(BC')/(BC)=(A'C')/(AC)=4/5`

 This justifies the construction.