Question

Draw a right triangle in which the sides (other than hypotenuse) are of lengths 4 cm and 3 cm. the construct another triangle whose sides are `5/3` times the corresponding sides of the given triangle. Give the justification of the construction.

Answer 1

It is given that sides other than hypotenuse are of lengths 4 cm and 3 cm. Clearly, these will be perpendicular to each other.

The required triangle can be drawn as follows.

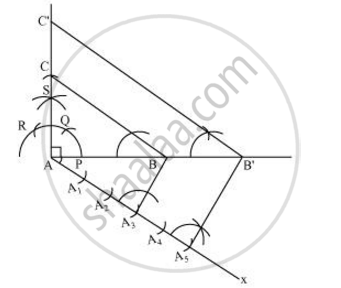
Step 1

Draw a line segment AB = 4 cm. Draw a ray SA making 90° with it.

Step 2

Draw an arc of 3 cm radius while taking A as its centre to intersect SA at C. Join BC. ΔABC is the required triangle.

Step 3

Draw a ray AX making an acute angle with AB, opposite to vertex C.

Step 4

Locate 5 points (as 5 is greater in 5 and 3), A₁, A₂, A₃, A₄, A₅, on line segment AX such that AA₁ = A₁A₂= A₂A₃ = A₃A₄ = A₄A₅.

Step 5

Join A₃B. Draw a line through A₅ parallel to A₃B intersecting extended line segment AB at B'.

Step 6

Through B', draw a line parallel to BC intersecting extended line segment AC at C'. ΔAB'C' is the required triangle.

Justification

The construction can be justified by proving that

`AB' =5/3 AB, B'C' = 5/3 BC, AC' = 5/3 AC`

In ΔABC and ΔAB'C',

∠ABC = ∠AB'C' (Corresponding angles)

∠BAC = ∠B'AC' (Common)

∴ ΔABC ∼ ΔAB'C' (AA similarity criterion)

`=> (AB)/(AB')=(BC)/(B'C') = (AC)/(AC') ...1`

In ΔAA₃B and ΔAA₅B',

∠A₃AB = ∠A₅AB' (Common)

∠AA₃B = ∠AA₅B' (Corresponding angles)

∴ ΔAA₃B ∼ ΔAA₅B' (AA similarity criterion)

`=> (AB)/(AB') = (`

`=>(AB)/(AB') = 3/5 ....2`

On comparing equations (1) and (2), we obtain

`(AB)/(AB') = (BC)/(B'C') = (AC)/(AC') = 3/5`

`=> AB' =5/3 AB, B'C' = 5/3 BC, AC' = 5/3 AC`

This justifies the construction.