Advertisements
Advertisements
Question
Equipotential surfaces ______.
- are closer in regions of large electric fields compared to regions of lower electric fields.
- will be more crowded near sharp edges of a conductor.
- will be more crowded near regions of large charge densities.
- will always be equally spaced.
Options
a, b and c
a, c and d
b, c and d
c and d
Advertisements
Solution
a, b and c
Explanation:
The density of the equipotential lines gives an idea about the magnitude of electric field. Higher the density, larger the field strength.
We know, the electric field intensity E and electric potential V are related as a, b and c
We know that on any two points of equipotential surface, potential difference is zero or of equal potential.
∵ `E = (-dV)/(dr)`
So the electric field intensity is inversely proportional to the separation between equipotential surfaces.
So equipotential surfaces are closer in regions of large electric. Thus, it verifies answer a
The electric field is larger near the sharp edge, due to larger charge density as a is very small
∵ `sigma = q/A`
So equipotential surfaces are closer or crowded. It verifies answer b.
As the electric field `E = (kq)/r^2` and potential or field decreases as size of the body increases or vice-versa (case of the earth), so the equipotential surfaces will be more crowded if the charge density `sigma = q/A` increases. It verifies the answer c.
As the equipotential surface depends on distance r by `E = (-dV)/r` and `V = (kq)/r`. Equipotential surface depends on charge density at that place which is different at a different place, so equipotential surface are not equispaced all over.
Hence the electric field intensity E is inversely proportional to the separation between equipotential surfaces. So, equipotential surfaces are closer in regions of large electric fields. As electric field intensities is large near sharp edges of charged conductor and near regions of large charge densities. Therefore, equipotential surfaces are closer at such places.
APPEARS IN
RELATED QUESTIONS
Draw a sketch of equipotential surfaces due to a single charge (-q), depicting the electric field lines due to the charge
A regular hexagon of side 10 cm has a charge 5 µC at each of its vertices. Calculate the potential at the centre of the hexagon.
Two charges 2 μC and −2 µC are placed at points A and B 6 cm apart.
- Identify an equipotential surface of the system.
- What is the direction of the electric field at every point on this surface?
Describe schematically the equipotential surfaces corresponding to
(a) a constant electric field in the z-direction,
(b) a field that uniformly increases in magnitude but remains in a constant (say, z) direction,
(c) a single positive charge at the origin, and
(d) a uniform grid consisting of long equally spaced parallel charged wires in a plane.
The top of the atmosphere is at about 400 kV with respect to the surface of the earth, corresponding to an electric field that decreases with altitude. Near the surface of the earth, the field is about 100 Vm−1. Why then do we not get an electric shock as we step out of our house into the open? (Assume the house to be a steel cage so there is no field inside!)
What is the geometrical shape of equipotential surfaces due to a single isolated charge?
Depict the equipotential surfaces for a system of two identical positive point charges placed a distance(d) apart?
Two identical point charges, q each, are kept 2m apart in the air. A third point charge Q of unknown magnitude and sign is placed on the line joining the charges such that the system remains in equilibrium. Find the position and nature of Q.
Draw the equipotential surfaces due to an electric dipole.
A particle of mass 'm' having charge 'q' is held at rest in uniform electric field of intensity 'E'. When it is released, the kinetic energy attained by it after covering a distance 'y' will be ______.
S1 and S2 are the two imaginary surfaces enclosing the charges +q and -q as shown. The electric flux through S1 and S2 are respectively ______.
The diagrams below show regions of equipotentials.
(i) |
(ii) |
(iii) |
(iv) |
A positive charge is moved from A to B in each diagram.
- The potential at all the points on an equipotential surface is same.
- Equipotential surfaces never intersect each other.
- Work done in moving a charge from one point to other on an equipotential surface is zero.
Equipotential surfaces ______.
Can two equipotential surfaces intersect each other?
Prove that a closed equipotential surface with no charge within itself must enclose an equipotential volume.
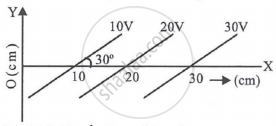
Equipotential surfaces are shown in figure. Then the electric field strength will be ______.
