Advertisements
Advertisements
प्रश्न
Equipotential surfaces ______.
- are closer in regions of large electric fields compared to regions of lower electric fields.
- will be more crowded near sharp edges of a conductor.
- will be more crowded near regions of large charge densities.
- will always be equally spaced.
पर्याय
a, b and c
a, c and d
b, c and d
c and d
Advertisements
उत्तर
a, b and c
Explanation:
The density of the equipotential lines gives an idea about the magnitude of electric field. Higher the density, larger the field strength.
We know, the electric field intensity E and electric potential V are related as a, b and c
We know that on any two points of equipotential surface, potential difference is zero or of equal potential.
∵ `E = (-dV)/(dr)`
So the electric field intensity is inversely proportional to the separation between equipotential surfaces.
So equipotential surfaces are closer in regions of large electric. Thus, it verifies answer a
The electric field is larger near the sharp edge, due to larger charge density as a is very small
∵ `sigma = q/A`
So equipotential surfaces are closer or crowded. It verifies answer b.
As the electric field `E = (kq)/r^2` and potential or field decreases as size of the body increases or vice-versa (case of the earth), so the equipotential surfaces will be more crowded if the charge density `sigma = q/A` increases. It verifies the answer c.
As the equipotential surface depends on distance r by `E = (-dV)/r` and `V = (kq)/r`. Equipotential surface depends on charge density at that place which is different at a different place, so equipotential surface are not equispaced all over.
Hence the electric field intensity E is inversely proportional to the separation between equipotential surfaces. So, equipotential surfaces are closer in regions of large electric fields. As electric field intensities is large near sharp edges of charged conductor and near regions of large charge densities. Therefore, equipotential surfaces are closer at such places.
APPEARS IN
संबंधित प्रश्न
Define an equipotential surface.
A regular hexagon of side 10 cm has a charge 5 µC at each of its vertices. Calculate the potential at the centre of the hexagon.
Two charges 2 μC and −2 µC are placed at points A and B 6 cm apart.
- Identify an equipotential surface of the system.
- What is the direction of the electric field at every point on this surface?
Describe schematically the equipotential surfaces corresponding to
(a) a constant electric field in the z-direction,
(b) a field that uniformly increases in magnitude but remains in a constant (say, z) direction,
(c) a single positive charge at the origin, and
(d) a uniform grid consisting of long equally spaced parallel charged wires in a plane.
The top of the atmosphere is at about 400 kV with respect to the surface of the earth, corresponding to an electric field that decreases with altitude. Near the surface of the earth, the field is about 100 Vm−1. Why then do we not get an electric shock as we step out of our house into the open? (Assume the house to be a steel cage so there is no field inside!)
The discharging current in the atmosphere due to the small conductivity of air is known to be 1800 A on an average over the globe. Why then does the atmosphere not discharge itself completely in due course and become electrically neutral? In other words, what keeps the atmosphere charged?
What are the forms of energy into which the electrical energy of the atmosphere is dissipated during a lightning?
(Hint: The earth has an electric field of about 100 Vm−1 at its surface in the downward direction, corresponding to a surface charge density = −10−9 C m−2. Due to the slight conductivity of the atmosphere up to about 50 km (beyond which it is good conductor), about + 1800 C is pumped every second into the earth as a whole. The earth, however, does not get discharged since thunderstorms and lightning occurring continually all over the globe pump an equal amount of negative charge on the earth.)
Draw equipotential surfaces:
(1) in the case of a single point charge and
(2) in a constant electric field in Z-direction. Why are the equipotential surfaces about a single charge not equidistant?
(3) Can electric field exist tangential to an equipotential surface? Give reason
Draw the equipotential surfaces due to an electric dipole. Locate the points where the potential due to the dipole is zero.
Two identical point charges, q each, are kept 2m apart in the air. A third point charge Q of unknown magnitude and sign is placed on the line joining the charges such that the system remains in equilibrium. Find the position and nature of Q.
Depict the equipotential surface due to
(i) an electric dipole,
(ii) two identical positive charges separated by a distance.
Find the amount of work done in rotating an electric dipole of dipole moment 3.2 x 10- 8Cm from its position of stable equilibrium to the position of unstable equilibrium in a uniform electric field if intensity 104 N/C.
A particle of mass 'm' having charge 'q' is held at rest in uniform electric field of intensity 'E'. When it is released, the kinetic energy attained by it after covering a distance 'y' will be ______.
The diagrams below show regions of equipotentials.
(i) |
(ii) |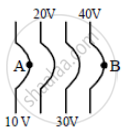
(iii) |
(iv) |
A positive charge is moved from A to B in each diagram.
Equipotential surfaces ______.
Which of the following is NOT the property of equipotential surface?
Equipotential surfaces are shown in figure. Then the electric field strength will be ______.
