Advertisements
Advertisements
प्रश्न
A regular hexagon of side 10 cm has a charge 5 µC at each of its vertices. Calculate the potential at the centre of the hexagon.
उत्तर
The given figure shows six equal amounts of charges, q, at the vertices of a regular hexagon.
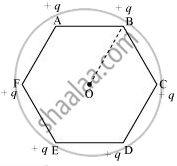
Where,
Charge, q = 5 µC = 5 × 10−6 C
Side of the hexagon, l = AB = BC = CD = DE = EF = FA = 10 cm
Distance of each vertex from centre O, d = 10 cm
Electric potential at point O,
`"V" = (6 xx "q")/(4piin_0"d")`
Where,
`in_0` = Permittivity of free space
`1/(4piin_0) = 9 xx 10^9 "NC"^-2 "m"^-2`
∴ `"V" = (6 xx 9 xx 10^9 xx 5 xx 10^-6)/0.1`
= 2.7 × 106 V
Therefore, the potential at the centre of the hexagon is 2.7 × 106 V.
APPEARS IN
संबंधित प्रश्न
The top of the atmosphere is at about 400 kV with respect to the surface of the earth, corresponding to an electric field that decreases with altitude. Near the surface of the earth, the field is about 100 Vm−1. Why then do we not get an electric shock as we step out of our house into the open? (Assume the house to be a steel cage so there is no field inside!)
Draw equipotential surfaces:
(1) in the case of a single point charge and
(2) in a constant electric field in Z-direction. Why are the equipotential surfaces about a single charge not equidistant?
(3) Can electric field exist tangential to an equipotential surface? Give reason
Draw the equipotential surfaces due to an electric dipole. Locate the points where the potential due to the dipole is zero.
Depict the equipotential surfaces for a system of two identical positive point charges placed a distance(d) apart?
Answer the following question.
Two identical point charges, q each, are kept 2m apart in the air. A third point charge Q of unknown magnitude and sign is placed on the line joining the charges such that the system remains in equilibrium. Find the position and nature of Q.
Draw the equipotential surfaces due to an electric dipole.
Answer the following question.
Write two important characteristics of equipotential surfaces.
Consider the following statements and select the correct statement(s).
- Electric field lines are always perpendicular to equipotential surface.
- No two equipotential surfaces can intersect each other.
- Electric field lines are in the direction of tangent to an equipotential surface.
Equipotentials at a great distance from a collection of charges whose total sum is not zero are approximately.
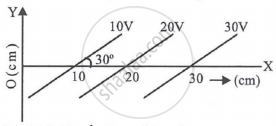
The diagrams below show regions of equipotentials.
(i) |
(ii)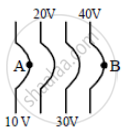 |
(iii) |
(iv) |
A positive charge is moved from A to B in each diagram.
- The potential at all the points on an equipotential surface is same.
- Equipotential surfaces never intersect each other.
- Work done in moving a charge from one point to other on an equipotential surface is zero.
Equipotential surfaces ______.
Can two equipotential surfaces intersect each other?
Find the equation of the equipotentials for an infinite cylinder of radius r0, carrying charge of linear density λ.
Draw equipotential surfaces for (i) an electric dipole and (ii) two identical positive charges placed near each other.
Equipotential surfaces are shown in figure. Then the electric field strength will be ______.