Advertisements
Advertisements
प्रश्न
Find the equation of the equipotentials for an infinite cylinder of radius r0, carrying charge of linear density λ.
उत्तर
To find the potential at distance r from the line consider the electric field. We note that from symmetry the field lines must be radially outward. Draw a cylindrical Gaussian surface of radius r and length l. Then
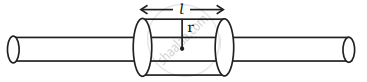
`oint E.dS = 1/ε_0 λ1`
Or `E_r.2pirl = 1/ε_0 λ1`
⇒ `E_r = lambda/(2piε_0r)`
Hence, if r0 is the radius,
`V(r) - V(r_0) = - int_(r_0)^r E.dl = λ/(2piε_0)ln r_0/r`
For a given V,
ln `r/r_0 = - (2piε_0)/λ [V(r) - V(r_0)]`
⇒ r = r0e –2πε0Vr0/λe + 2πε0V(r)/λ
The equipotential surfaces are cylinders of radius r = r0e –2πε0[V(r) – V(r0)]/λ
APPEARS IN
संबंधित प्रश्न
Define an equipotential surface.
What are the forms of energy into which the electrical energy of the atmosphere is dissipated during a lightning?
(Hint: The earth has an electric field of about 100 Vm−1 at its surface in the downward direction, corresponding to a surface charge density = −10−9 C m−2. Due to the slight conductivity of the atmosphere up to about 50 km (beyond which it is good conductor), about + 1800 C is pumped every second into the earth as a whole. The earth, however, does not get discharged since thunderstorms and lightning occurring continually all over the globe pump an equal amount of negative charge on the earth.)
Draw the equipotential surfaces due to an electric dipole. Locate the points where the potential due to the dipole is zero.
Why is there no work done in moving a charge from one point to another on an equipotential surface?
Depict the equipotential surfaces for a system of two identical positive point charges placed a distance(d) apart?
Answer the following question.
Two identical point charges, q each, are kept 2m apart in the air. A third point charge Q of unknown magnitude and sign is placed on the line joining the charges such that the system remains in equilibrium. Find the position and nature of Q.
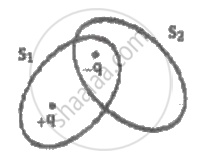
S1 and S2 are the two imaginary surfaces enclosing the charges +q and -q as shown. The electric flux through S1 and S2 are respectively ______.
- The potential at all the points on an equipotential surface is same.
- Equipotential surfaces never intersect each other.
- Work done in moving a charge from one point to other on an equipotential surface is zero.
Equipotential surfaces ______.
- are closer in regions of large electric fields compared to regions of lower electric fields.
- will be more crowded near sharp edges of a conductor.
- will be more crowded near regions of large charge densities.
- will always be equally spaced.
What is meant by an equipotential surface?
