Advertisements
Advertisements
प्रश्न
Find the equation of the equipotentials for an infinite cylinder of radius r0, carrying charge of linear density λ.
Advertisements
उत्तर
To find the potential at distance r from the line consider the electric field. We note that from symmetry the field lines must be radially outward. Draw a cylindrical Gaussian surface of radius r and length l. Then
`oint E.dS = 1/ε_0 λ1`
Or `E_r.2pirl = 1/ε_0 λ1`
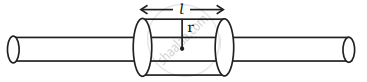
⇒ `E_r = lambda/(2piε_0r)`
Hence, if r0 is the radius,
`V(r) - V(r_0) = - int_(r_0)^r E.dl = λ/(2piε_0)ln r_0/r`
For a given V,
ln `r/r_0 = - (2piε_0)/λ [V(r) - V(r_0)]`
⇒ r = r0e –2πε0Vr0/λe + 2πε0V(r)/λ
The equipotential surfaces are cylinders of radius r = r0e –2πε0[V(r) – V(r0)]/λ
APPEARS IN
संबंधित प्रश्न
Draw a sketch of equipotential surfaces due to a single charge (-q), depicting the electric field lines due to the charge
Describe schematically the equipotential surfaces corresponding to
(a) a constant electric field in the z-direction,
(b) a field that uniformly increases in magnitude but remains in a constant (say, z) direction,
(c) a single positive charge at the origin, and
(d) a uniform grid consisting of long equally spaced parallel charged wires in a plane.
The top of the atmosphere is at about 400 kV with respect to the surface of the earth, corresponding to an electric field that decreases with altitude. Near the surface of the earth, the field is about 100 Vm−1. Why then do we not get an electric shock as we step out of our house into the open? (Assume the house to be a steel cage so there is no field inside!)
What are the forms of energy into which the electrical energy of the atmosphere is dissipated during a lightning?
(Hint: The earth has an electric field of about 100 Vm−1 at its surface in the downward direction, corresponding to a surface charge density = −10−9 C m−2. Due to the slight conductivity of the atmosphere up to about 50 km (beyond which it is good conductor), about + 1800 C is pumped every second into the earth as a whole. The earth, however, does not get discharged since thunderstorms and lightning occurring continually all over the globe pump an equal amount of negative charge on the earth.)
Draw the equipotential surfaces due to an electric dipole.
Depict the equipotential surface due to
(i) an electric dipole,
(ii) two identical positive charges separated by a distance.
Statement - 1: For practical purpose, the earth is used as a reference at zero potential in electrical circuits.
Statement - 2: The electrical potential of a sphere of radius R with charge Q uniformly distributed on the surface is given by `Q/(4piepsilon_0R)`.
A particle of mass 'm' having charge 'q' is held at rest in uniform electric field of intensity 'E'. When it is released, the kinetic energy attained by it after covering a distance 'y' will be ______.
S1 and S2 are the two imaginary surfaces enclosing the charges +q and -q as shown. The electric flux through S1 and S2 are respectively ______.
Assertion: Electric field is discontinuous across the surface of a spherical charged shell.
Reason: Electric potential is continuous across the surface of a spherical charged shell.
Equipotentials at a great distance from a collection of charges whose total sum is not zero are approximately.
The work done to move a charge along an equipotential from A to B ______.
- cannot be defined as `- int_A^B E.dl`
- must be defined as `- int_A^B E.dl`
- is zero.
- can have a non-zero value.
Draw equipotential surfaces for (i) an electric dipole and (ii) two identical positive charges placed near each other.
What is meant by an equipotential surface?
