Advertisements
Advertisements
Question
Figure shows a collar B which moves upwards with constant velocity of 1.5 m/s.At the instant when θ=50o.Determine :
(i)The angular velocity of rod pinned at B and freely resting at A against 25o sloping ground.
(ii)The velocity of end A of the rod.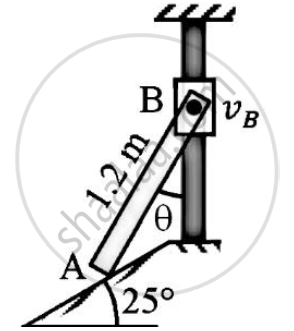
Solution
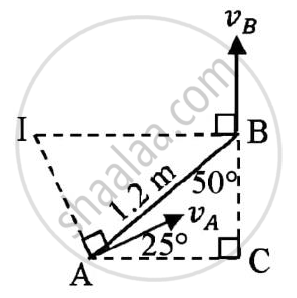
ICR is shown in the given figure
BY USING GEOMETRY:
In △ABC
∠ABC = 50
∠ACB = 90
∠BAC = 40
∠CAV = 25
∠BAV = 40 – 25 = 15
IA ⊥ VA
∠IAB = 90 – 15 = 75
∠IBA = 90 – 50 = 40
In △IBA
∠BIA = 180 – 75 = 65
In △IBA
AB=1.2 m
APPLYING SINE RULE
`(AB)/(sin I)=(IB)/sinA=(IA)/(sinB)`
`1.2/sin65=(IB)/(sinA)=(IA)/sin40`
IB=1.2789 m
IA=0.8511 m
Assume ωAB be the angular velocity of AB
ωAB = `V_v/r=V_B/(IB)=1.5/1.2789` = 1.1728 rad/s
vA = r x AB = IA x ωAB = 0.8511 x 1.7288 = 0.99825 m/s
Angular velocity of rod AB= 1.1728 rads (Anti-clockwise)
Instantaneous velocity of A = 0.9982 m/s( 25° in first quadrant)
APPEARS IN
RELATED QUESTIONS
From (v-t) diagram find (1) Distance travelled in 10 second.
(2) Total distance travelled in 50 second.
(3) Retardation
Determine the speed at which the basket ball at A must be thrown at an angle 30o so that if makes it to the basket at B.
Also find at what speed it passes through the hoop.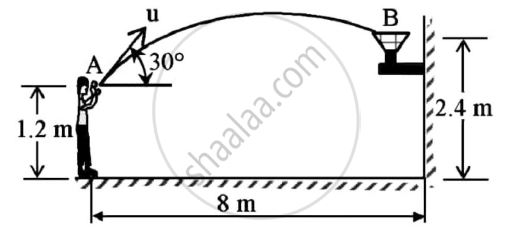
Find an expression for maximum range of a particle which is projected with an initial velocity of ‘u’ inclined at an angle of ‘β’ with the horizontal.
