Advertisements
Advertisements
Question
Figure shows a square loop of edge a made of a uniform wire. A current i enters the loop at the point A and leaves it at the point C. Find the magnetic field at the point P which is on the perpendicular bisector of AB at a distance a/4 from it.
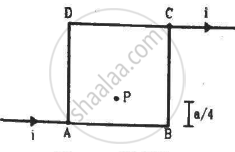
Solution
B at P due to AD = \[\frac{\mu_0}{4\pi} . \frac{i}{2} . \frac{4}{d^2} . a\left[ \frac{\left( \frac{a}{2} \right)}{\sqrt{\left( \frac{a}{2} \right)^2 + \left( \frac{a}{4} \right)^2}} + \frac{\left( \frac{a}{2} \right)}{\sqrt{\left( \frac{a}{2} \right)^2 + \left( \frac{3a}{4} \right)^2}} \right]\] along•
\[\frac{\mu_0 i}{4\pi a}\left[ \frac{\left( \frac{a}{2} \right)}{\sqrt{\left( \frac{a}{2} \right)^2 + \left( \frac{a}{4} \right)^2}} + \frac{\left( \frac{a}{2} \right)}{\sqrt{\left( \frac{a}{2} \right)^2 + \left( \frac{3a}{4} \right)^2}} \right]\] along•
B at P due to AC= \[\frac{\mu_0}{4\pi} . \frac{i}{2} . \frac{16}{9 a^2} . a . 2\left[ \frac{\left( \frac{3a}{4} \right)}{\sqrt{\left( \frac{3a}{4} \right)^2 + \left( \frac{a}{2} \right)^2}} \right]\]
\[= \frac{4 \mu_0 i}{9\pi a}\left[ \frac{\left( \frac{3a}{4} \right)}{\sqrt{\left( \frac{a}{4} \right)^2 + \left( \frac{3a}{2} \right)^2}} \right]\] along•
B at P due to AB = \[\frac{\mu_0}{4\pi} . \frac{i}{2} . \frac{16}{9 a^2} . a . 2\left[ \frac{\left( \frac{3a}{4} \right)}{\sqrt{\left( \frac{3a}{4} \right)^2 + \left( \frac{a}{2} \right)^2}} \right]\] along•
B at P due to BC = \[\frac{\mu_0}{4\pi} . \frac{i}{2} . \frac{4}{a^2} . a . \left[ \frac{\left( \frac{a}{2} \right)}{\sqrt{\left( \frac{a}{2} \right)^2 + \left( \frac{a}{4} \right)^2}} + \frac{\left( \frac{a}{2} \right)}{\sqrt{\left( \frac{a}{2} \right)^2 + \left( \frac{3a}{4} \right)^2}} \right]\] along•
\[ = \frac{4 \mu_0 i}{\pi a}\frac{1}{4}\left[ \frac{1}{\sqrt{\frac{1}{4} + \frac{1}{16}}} \right] - \frac{4 \mu_0 i}{9\pi a} . \frac{3}{4}\left[ \frac{1}{\sqrt{\frac{1}{4} + \frac{9}{16}}} \right]\]
APPEARS IN
RELATED QUESTIONS
A square coil of side 10 cm consists of 20 turns and carries a current of 12 A. The coil is suspended vertically and the normal to the plane of the coil makes an angle of 30° with the direction of a uniform horizontal magnetic field of magnitude 0.80 T. What is the magnitude of torque experienced by the coil?
In a chamber, a uniform magnetic field of 6.5 G (1 G = 10–4 T) is maintained. An electron is shot into the field with a speed of 4.8 × 106 m s−1 normal to the field. Explain why the path of the electron is a circle. Determine the radius of the circular orbit. (e = 1.5 × 10–19 C, me = 9.1 × 10–31 kg)
A rectangular loop of wire of size 4 cm × 10 cm carries a steady current of 2 A. A straight long wire carrying 5 A current is kept near the loop as shown. If the loop and the wire are coplanar, find
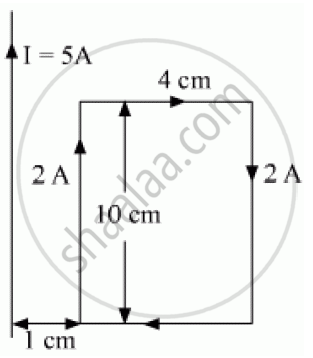
(i) the torque acting on the loop and
(ii) the magnitude and direction of the force on the loop due to the current carrying wire.
A rectangular loop of wire of size 2 cm × 5 cm carries a steady current of 1 A. A straight long wire carrying 4 A current is kept near the loop as shown. If the loop and the wire are coplanar, find (i) the torque acting on the loop and (ii) the magnitude and direction of the force on the loop due to the current carrying wire.

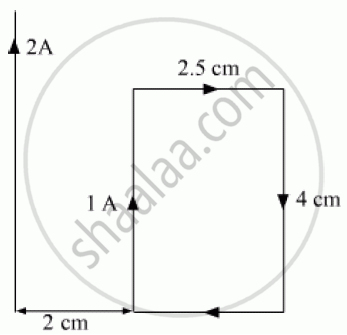
A rectangular loop of wire of size 2.5 cm × 4 cm carries a steady current of 1 A. A straight wire carrying 2 A current is kept near the loop as shown. If the loop and the wire are coplanar, find the (i) torque acting on the loop and (ii) the magnitude and direction of the force on the loop due to the current carrying wire.
A rectangular loop of size l × b carrying a steady current I is placed in a uniform magnetic field `vecB`. Prove that the torque `vectau`acting on the loop is give by `vectau =vecm xx vecB,`where `vecm` is the magnetic moment of the loop.
A magnetised needle of magnetic moment 4.8 × 10−2 JT−1 is placed at 30° with the direction of uniform magnetic field of magnitude 3 × 10−2 T. Calculate the torque acting on the needle.
Find the magnetic field B at the centre of a rectangular loop of length l and width b, carrying a current i.
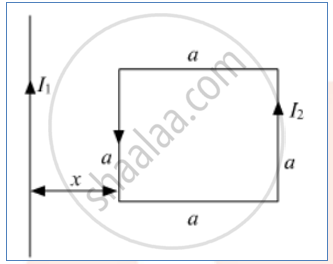
A square loop of side 'a' carrying a current I2 is kept at distance x from an infinitely long straight wire carrying a current I1 as shown in the figure. Obtain the expression for the resultant force acting on the loop.
A planar loop of rectangular shape is moved within the region of a uniform magnetic field acting perpendicular to its plane. What is the direction and magnitude of the current induced in it?
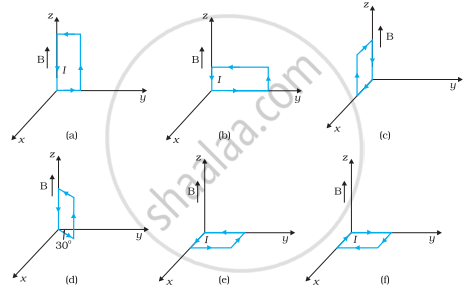
A uniform magnetic field of 3000 G is established along the positive z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A. What is the torque on the loop in the different cases shown in fig.? What is the force on each case? Which case corresponds to stable equilibrium?
A rectangular conducting loop consists of two wires on two opposite sides of length l joined together by rods of length d. The wires are each of the same material but with cross-sections differing by a factor of 2. The thicker wire has a resistance R and the rods are of low resistance, which in turn are connected to a constant voltage source V0. The loop is placed in uniform a magnetic field B at 45° to its plane. Find τ, the torque exerted by the magnetic field on the loop about an axis through the centres of rods.
- Assertion (A): The deflecting torque acting on a current-carrying loop is zero when its plane is perpendicular to the direction of the magnetic field.
- Reason (R): The deflecting torque acting on a loop of the magnetic moment `vecm` in a magnetic field `vecB` is given by the dot product of `vecm` and `vecB`.
