Question

Find `sin  x/2, cos  x/2 and tan  x/2` of the following:

`cos x =  -1/3`, x in quadrant III

Answer 1

 

Here, x is in quadrant III

i.e, `pi < x < (3x)/2`

⇒ `pi/2 < x/2 < (3pi)/4`

Therefore, cos `x/2` and tan `x/2` are negative, while sin `x/2` is positive.

It is given that cos x = - `1/3`

`cos x = 1 - 2 sin^2  x/2`

⇒ `sin^2  x/2 = (1 - cosx)/2`

⇒ `sin^2   x/2 =  (1 - (1/3)/2 = 1 + 1/3)/2 = (4/3)/2 = 2/3`

⇒ sin `x/2 = sqrt2/sqrt3` `[∵ sin  x/2 "is positive"]`

∴ sin `x/2 = sqrt2/sqrt3 xx sqrt3/sqrt3 = sqrt6/3`

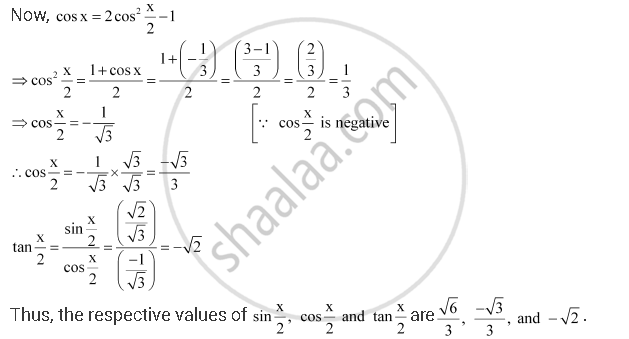
Now, cos x = `2cos^2  x/2 -1 ` 

⇒ `cos^2  x/2 = 1 +cosx/2 = 1+ (- 1/3)/2 = ((3-1) /3)/2 = (2/3)/2 = 1/3`

⇒ `cos  x/2 = - 1/sqrt3`   `[∵ cos  x/2  "is negative"]`

∴ `cos  x/2 = - 1/sqrt3 xx sqrt3/sqrt3 = (sqrt -3)/3`

`tan  x/2 = (sin  x/2 = (sqrt2/sqrt3))/(cos  x/2 (-1/sqrt3)) = - sqrt2` 

Thus, the corresponding values of `sin  x/2, cos  x/2 and tan  x/2 and sqrt6/3, (-sqrt3)/3 and -sqrt2`.