Advertisements
Advertisements
Question
Find the angle of incidence at face AB so that the emergent ray grazes along the face AC.
Solution
from snell's law we know that
`sini/sinr =μ_(12)`
For the emergent ray to graze at the face AC, the angle of refraction should be 90°.
So, applying snell's law at face AC, we get `(sini_(AC)/sinr_(AC) )= μ_(21)`
`⇒ (sin i_(AC)/sin 90^@)=1/sqrt2 or i_(AC)= 45°.`
From geometry we can see that angle of refraction at face AB is 15°.
So applying snell's law we get `(sin i_(AB)/sin r_(AB))= μ_(12)`
`or sin i_(AB) = sin r_(AB)×μ_(12)`
`or i_(AB) =sin^-1(sqrt2sin15°)`
RELATED QUESTIONS
At what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
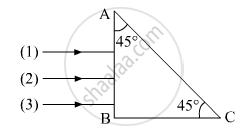
Three rays (1, 2, 3) of different colours fall normally on one of the sides of an isosceles right angled prism as shown. The refractive index of prism for these rays is 1.39, 1.47 and 1.52 respectively. Find which of these rays get internally reflected and which get only refracted from AC. Trace the paths of rays. Justify your answer with the help of necessary calculations.
Can you ever have a situation in which a light ray goes undeviated through a prism?
A flint glass prism and a crown glass prism are to be combined in such a way that the deviation of the mean ray is zero. The refractive index of flint and crown glasses for the mean ray are 1.620 and 1.518 respectively. If the refracting angle of the flint prism is 6.0°, what would be the refracting angle of the crown prism?
A small object is embedded in a glass sphere (μ = 1.5) of radius 5.0 cm at a distance 1.5 cm left to the centre. Locate the image of the object as seen by an observer standing (a) to the left of the sphere and (b) to the right of the sphere.
The refractive index of a prism whose angle A = 60° is `sqrt2`. Then the angle of minimum deviation δm will be ______.
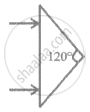
An isosceles prism of angle 120° has a refractive index 1.44. Two parallel monochromatic rays enter the prism parallel to each other in air as shown. The rays emerge from the opposite faces:
A ray PQ is incident normally on the face AB of a triangular prism of refracting angle 60° as shown in figure. The prism is made of a transparent material of refractive index `2/sqrt(3)`. Trace the path of the ray as it passes through the prism. Calculate the angle of emergence and the angle of deviation.
A ray of light when incident upon a thin prism suffers a minimum deviation of 39°. If the shaded half portion of the prism is removed, then the same ray will ______.
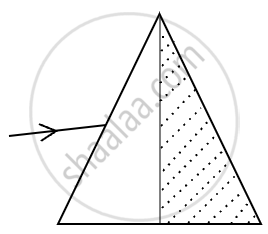
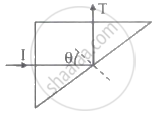
A triangular prism of glass is shown in the figure. A ray incident normally to one face is totally internally reflected. If θ is 45°, then the index of refraction of the glass is ______.