Question

Find the current through the 10 Ω resistor shown in the figure.

Answer 1

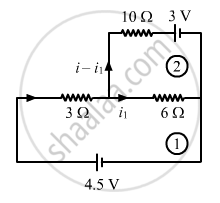
Applying KVL in loop 1, we get:-

3i + 6i₁ = 4.5 ...............(1)

Applying KVL in loop 2, we get:-

\[\left( i - i_1 \right)10 + 3 - 6 i_1 = 0\]

\[10i - 16 i_1 = - 3 ..............(2)\]

Multiplying equation (1) by 10 and (2) by 3 and then, subtracting (2) from (1), we get:-

\[- 108 i_1 = - 54\]

\[ \Rightarrow i_1 = \frac{54}{108} = \frac{1}{2} = 0 . 5\]

Substituting the value of i₁ in (1), we get:-

\[3i + 6 \times \frac{1}{2} - 4 . 5 = 0\]

\[3i - 1 . 5 = 0\]

\[ \Rightarrow i = \frac{1 . 5}{3} = 0 . 5\]

So, current flowing through the 10 Ω resistor = i - i₁ = 0.5 - 0.5 = 0 A