Question

Find the area of the ellipse `x^2/4 + y^2/25 = 1`

Answer 1

By the symmetry of the ellipse required area of the ellipse is 4 times the area of the region OPQO: For the regioN the limits of integration are x= Oandx= 2,

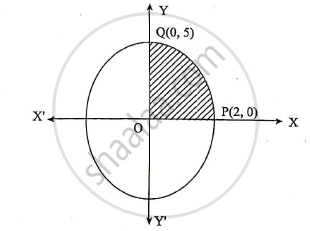
From the equation of the ellispe 

`x^2/4 + y^2/25 = 1`

`y^2/25 = 1 - x^2/4`

`y^2 = 25 (1 - x^2/4)`
        = 25 `((4 - x^2)/4)`
        = `25/4 (4 - x^2)`

y = `5/2 sqrt(4 - x^2)`

Now A = 4`int_0^2 y dx`

A = 4`int_0^2 5/2 sqrt(4 - x^2)` dx

= `20/2 int_0^2 sqrt(4 - x^2)` dx

`= 10 [x/2 sqrt(4 - x^2) + 4/2 (sin^-1 x/2)]_0^2        ...[∵ int sqrt(a^2-x^2) dx = x/2 sqrt(a^2 - x^2) + a^2/1 sin^-1(x/a)]`  

`=10 [{2/2 sqrt(4 - 4) + 2 sin^-1 (1)}- {0/2 sqrt(4-0) + 2 sin^-1(0)}]        ...[∵ sin^-1 (1)= pi/2,sin^-1(0)=0]`

= 10 [2 sin^-1 (1)]

= `20 (π/2)`

= 10 π sq. units.