Advertisements
Advertisements
Question
Find the central angle of the sectors whose measures are given below. `(π = 22/7)`
area = 462 cm2, r = 21 cm
Solution
area = 462 cm2, r = 21 cm
Radius of the Sector = 21 cm
Area of the sector = 462 cm2
`"lr"/2` = 462
`("l" xx 21)/2` = 462
l = `(462 xx 2)/21`
l = 22 × 2
Length of the arc l = 44 cm
`(theta^circ)/(360^circ) xx 2pi"r"` = 44 cm
`(theta^circ)/(360^circ) xx 2 xx 22/7 xx 21` = 44 cm
θ° = `(44 xx 360 xx 7)/(2 xx 22 xx 21)`
θ° = 120°
∴ Central angle of the sector = 120°
APPEARS IN
RELATED QUESTIONS
Some arcs are shown in the circle with centre ‘O’. Write the names of the minor arcs, major arcs, and semicircular arcs from among them.

In a circle with centre O, the measure of a minor arc is 110°. What is the measure of the major arc PYQ?
Find the central angle of the shaded sectors (each circle is divided into equal sectors).
| Sectors | 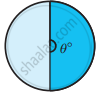 |
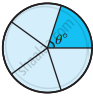 |
 |
 |
| Central angle of each sector (θ°) |
Find the central angle of the sectors whose measures are given below. `(π = 22/7)`
length of the arc = 44 m, r = 35 m
