Advertisements
Advertisements
Question
Find the slope of the lines passing through the given point.
C (5, –2) , D (7, 3)
Solution
Let, x1 = 5, y1 = - 2, x2 = 7, y2 = 3
∴ Slope of line CD = `(y_2 - y_1)/(x_2 - x_1)`
∴ Slope of line CD = `[3 - (- 2)]/[7 - 5]`
∴ Slope of line CD = `[3 + 2]/[7 - 5]`
∴ Slope of line CD = `5/2`
APPEARS IN
RELATED QUESTIONS
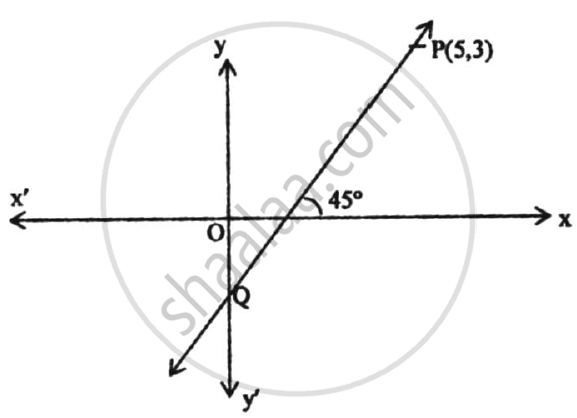
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
A(5, 4), B(−3, −2) and C(1, −8) are the vertices of a triangle ABC. Find:
- the slope of the altitude of AB,
- the slope of the median AD and
- the slope of the line parallel to AC.
The points (K, 3), (2, −4) and (−K + 1, −2) are collinear. Find K.
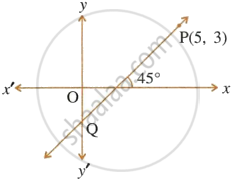
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
Find the value of p if the lines, whose equations are 2x – y + 5 = 0 and px + 3y = 4 are perpendicular to each other.
Determine whether the following point is collinear.
D(–2, –3), E(1, 0), F(2, 1)
If A(1, –1), B(0, 4), C(–5, 3) are vertices of a triangle then find the slope of each side.
Fill in the blank using correct alternative.
Seg AB is parallel to Y-axis and coordinates of point A are (1,3) then co–ordinates of point B can be ........ .
Find the slope of a line passing through the given pair of points (2,5) and (-1,8)
Find the slope of a line passing through the given pair of points (-5,-1) and (-9,-7)
Find the slope of a line parallel to the given line x +3y = 7
Find the slope and the y-intercept of the following line x - 2 = `(5 - 3"y")/2`
Find the slope of a line passing through the point A (-2,1), B (0,3).
The line through A (- 2, 3) and B (4, b) is perpendicular to the line 2a – 4y = 5. Find the value of b.
Show that the line joining (2, – 3) and (- 5, 1) is:
(i) Parallel to line joining (7, -1) and (0, 3).
(ii) Perpendicular to the line joining (4, 5) and (0, -2).
With out Pythagoras theorem, show that A(4, 4), B(3, 5) and C(-1, -1) are the vertices of a right angled.
If the lines kx – y + 4 = 0 and 2y = 6x + 7 are perpendicular to each other, find the value of k.
