Advertisements
Advertisements
Question
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
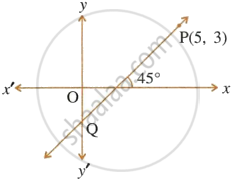
Solution
i. The equation of the y-axis is x = 0
Given that the required line through P(5, 3)
Intersects the y-axis at Q and the angle of inclination is 45°
Therefore slope of the line PQ = tan 45° = 1
ii. The equation of a line passing through the point
A(x1, y1) with slope ‘m’ is
y – y1 = m(x – x1)
Therefore, the equation of the line passing through the point P(5, 3) with slope 1 is
y – 3 = 1 × (x – 5)
`=>` y – 3 = x – 5
`=>` x – y = 2
iii. From subpart (ii), the equation of the line PQ
Is x – y = 2
Given that the line intersects with the y-axis, x = 0
Thus, substituting x = 0 in the equation x – y = 2
We have, 0 – y = 2
`=>` y = −2
Thus, the coordinates point of intersection Q are q(0, −2)
APPEARS IN
RELATED QUESTIONS
Find the slope of the line passing through the points A(2, 3) and B(4, 7).
A and B are two points on the x-axis and y-axis respectively. P (2, −3) is the midpoint of AB. Find the:
(1) coordinates of A and B
(2) slope of line AB.
(3) an equation of line AB.

Find the slope of the line parallel to AB if : A = (−2, 4) and B = (0, 6)
Find the slope of the lines passing through the given point.
C (5, –2) , D (7, 3)
Determine whether the following point is collinear.
L(2, 5), M(3, 3), N(5, 1)
Find k, if PQ || RS and P(2, 4), Q (3, 6), R(3, 1), S(5, k).
Find the slope of a line parallel to the given line 3x-2y = 5
Find the slope and the y-intercept of the following line 4y = 5x - 8
Find slope of a line passing through the points A(3, 1) and B(5, 3).
Find the slope of a line having inclination 60°.
