Advertisements
Advertisements
प्रश्न
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
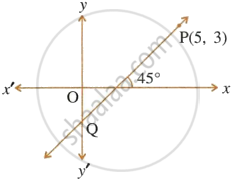
उत्तर
i. The equation of the y-axis is x = 0
Given that the required line through P(5, 3)
Intersects the y-axis at Q and the angle of inclination is 45°
Therefore slope of the line PQ = tan 45° = 1
ii. The equation of a line passing through the point
A(x1, y1) with slope ‘m’ is
y – y1 = m(x – x1)
Therefore, the equation of the line passing through the point P(5, 3) with slope 1 is
y – 3 = 1 × (x – 5)
`=>` y – 3 = x – 5
`=>` x – y = 2
iii. From subpart (ii), the equation of the line PQ
Is x – y = 2
Given that the line intersects with the y-axis, x = 0
Thus, substituting x = 0 in the equation x – y = 2
We have, 0 – y = 2
`=>` y = −2
Thus, the coordinates point of intersection Q are q(0, −2)
APPEARS IN
संबंधित प्रश्न
Find the value(s) of k so that PQ will be parallel to RS. Given : P(3, −1), Q(7, 11), R(−1, −1) and S(1, k)
Determine x so that the slope of the line through (1, 4) and (x, 2) is 2.
Find the slope of the line which is parallel to x + 2y + 3 = 0
Angles made by the line with the positive direction of X–axis is given. Find the slope of these line.
90°
Determine whether the following point is collinear.
A(–4, 4), \[K\left( - 2, \frac{5}{2} \right),\] N (4, –2)
If A(1, –1), B(0, 4), C(–5, 3) are vertices of a triangle then find the slope of each side.
Find the slope of a line passing through the given pair of points (-5,-1) and (-9,-7)
Find the slope of a line parallel to the given line 5x + 2y = 11
Find the slope of a line passing through the following pair of points
(5pq,p2q) and (5qr,qr2)
Find the image of a point (-1, 2) in the line joining (2, 1) and (- 3, 2).
