Question

Find the volume of the solid generated, when the area between ellipse 4x² + 9y² = 36 and the chord AB, with A (3, 0), B (0, 2), is revolved about X-axis.

Answer 1

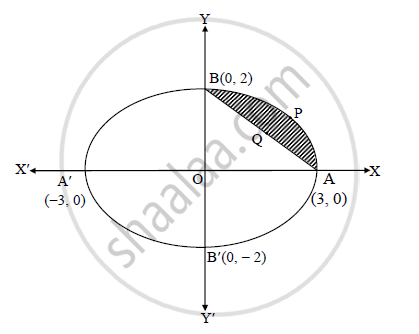
Given equation of ellipse is 4x² + 9y² = 36

`:. y^2 = 4/9(9 - x^2)`.....(i)

and equation of the chord is `x/3 + y/2 = 1`

∴ 2x + 3y = 6

∴ 3y = 6 − 2x

∴ y = 2 − `2/3x`

∴ `y^2 = (2 - 2/3 x)^2 = 4 - 8/3x + 4/9 x^2 `  ....(ii)

Required solid is obtained by revolving the shaded region about the X-axis between x = 0 and x = 3.

Let V₁ = volume of solid obtained by revolving the region OAPBO under the ellipse

V₂
= volume of solid obtained by revolving the region OAQBO under the chord AB.

∴ V = V₁ − V₂