Advertisements
Advertisements
Question
Find x.
`((-6)/7)^(x - 7) = 1`
Solution
We have, `((-6)/7)^(x - 7) = 1`
Using law of exponents, x0 = 1 ...[∵ a is non-zero integer]
Then, `((-6)/7)^(x - 7) = 1`
It is possible only, if x = 7.
So, `((-6)/7)^(7 - 7) = 1`
⇒ `((-6)/7)^0 = 1` ...[∵ a0 = 1]
Hence, x = 7
APPEARS IN
RELATED QUESTIONS
If x be any integer different from zero and m be any positive integer, then x–m is equal to ______.
`(1/10)^0` is equal to ______.
Solve the following:
2–2 × 2–3
An insect is on the 0 point of a number line, hopping towards 1. She covers half the distance from her current location to 1 with each hop. So, she will be at `1/2` after one hop, `3/4` after two hops, and so on.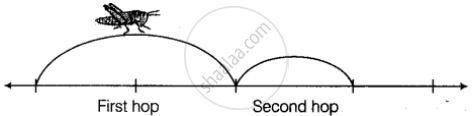
Will the insect ever get to 1? Explain.
Use the properties of exponents to verify that statement is true.
`1/4 (2^n) = 2^(n - 2)`
Find a single repeater machine that will do the same work as hook-up.
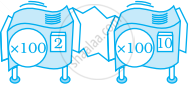
Supply the missing information for diagram.
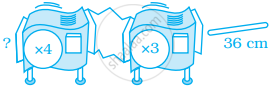
If possible, find a hook-up of prime base number machine that will do the same work as the given stretching machine. Do not use (× 1) machines.

Find a repeater machine that will do the same work as a `(xx 1/8)` machine.
Find x.
`(- 1/7)^-5 ÷ (- 1/7)^-7 = (-7)^x`
