Advertisements
Advertisements
Question
"For any charge configuration, equipotential surface through a point is normal to the electric field." Justify.
Solution
We know that the work done (W) in moving a test charge along an equipotential surface is zero. This is because an equipotential surface is a surface with a constant value of potential
at all the points on the surface.
∴ W = Fs cosθ = 0
Here, F is the electric force and s is the magnitude of displacement of the charge.
For non-zero displacement, this is possible only when cosθ is equal to 0.
i.e. cosθ = 0
⇒θ = 90°
Thus, the force acting on the point charge is perpendicular to the equipotential surface. We know that the lines of force or the electric field lines indicate the direction of electric force on a charge. Thus, for any charge configuration, equipotential surface through a point is normal to the electric field.
APPEARS IN
RELATED QUESTIONS
A 36 cm long sonometer wire vibrates with frequency of 280 Hz in fundamental mode, when it is under tension of 24.5 N. Calculate linear density of the material of wire.
Explain why, for a charge configuration, the equipotential surface through a point is normal to the electric field at that point
A point charge causes an electric flux of −1.0 × 103 Nm2/C to pass through a spherical Gaussian surface of 10.0 cm radius centred on the charge.
- If the radius of the Gaussian surface were doubled, how much flux would pass through the surface?
- What is the value of the point charge?
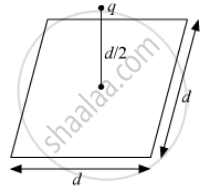
A point charge q is at a distance of d/2 directly above the centre of a square of side d, as shown the figure. Use Gauss' law to obtain the expression for the electric flux through the square.
Use Gauss' law to derive the expression for the electric field `(vecE)` due to a straight uniformly charged infinite line of charge density λ C/m.
Draw a graph to show the variation of E with perpendicular distance r from the line of charge.
Find the work done in bringing a charge q from perpendicular distance r1 to r2 (r2 > r1)
Consider a sphere of radius R with charge density distributed as
ρ(r) = kr for r ≤ R
= 0 for r > R
- Find the electric field at all points r.
- Suppose the total charge on the sphere is 2e where e is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.
A solid metal sphere of radius R having charge q is enclosed inside the concentric spherical shell of inner radius a and outer radius b as shown in the figure. The approximate variation of the electric field `vecE` as a function of distance r from centre O is given by ______.

