Advertisements
Advertisements
Question
A point charge q is at a distance of d/2 directly above the centre of a square of side d, as shown the figure. Use Gauss' law to obtain the expression for the electric flux through the square.
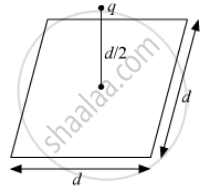
Solution
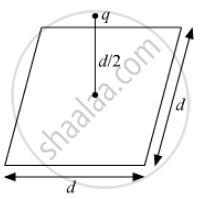
If we imagine a cube of side d, then this point charge q will be at the centre of the cube.
Now, the total electric flux due to this charge will pass evenly through the six faces of the cube.
So, the electric flux through one face will be equal to 1/6 of the total electric flux due to this charge.
Now, the total electric flux generated by the charge will be given by Gauss's law as :
`triangle phi = vecE.vec(triangleS)` = q/6∈0
So, the electric flux through the square = q/6∈∘">∈∘
APPEARS IN
RELATED QUESTIONS
"For any charge configuration, equipotential surface through a point is normal to the electric field." Justify.
Obtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss’s law. [Hint: Use Coulomb’s law directly and evaluate the necessary integral.]
Use Gauss' law to derive the expression for the electric field `(vecE)` due to a straight uniformly charged infinite line of charge density λ C/m.
The electric field intensity outside the charged conducting sphere of radius ‘R’, placed in a medium of permittivity ∈ at a distance ‘r’ from the centre of the sphere in terms of surface charge density σ is
A closed surface in vacuum encloses charges –q and +3q. The total electric flux emerging out of the surface is :
State Gauss’Law.
Two wires A and B of the same material and of equal length with the radii in the ratio 1 : 2 are subjected to identical loads. If the length of A increases by 8 mm, then the increase in length of B is:
Through two parallel wires A and B, 10A and 2A of currents are passed respectively in opposite directions. If the wire A is infinitely long and the length of the wire B is 2m, then force on the conductor B, which is situated at 10 cm distance from A, will be:
Consider a sphere of radius R with charge density distributed as
ρ(r) = kr for r ≤ R
= 0 for r > R
- Find the electric field at all points r.
- Suppose the total charge on the sphere is 2e where e is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.
- Obtain the expression for the electric field intensity due to a uniformly charged spherical shell of radius R at a point distant r from the centre of the shell outside it.
- Draw a graph showing the variation of electric field intensity E with r, for r > R and r < R.
