Advertisements
Advertisements
Question
State Gauss’Law.
Solution
Gauss’Law :
The electric flux `(phi_"E")` through any closed surface is equal to `1/in_0` times the 'net' change q enclosed by the surface.
`phi_"E" = oint vec"E" "d" vec"A" = "q"/in_0`
∈0 = Permittivity of free space.
APPEARS IN
RELATED QUESTIONS
Explain why, for a charge configuration, the equipotential surface through a point is normal to the electric field at that point
A point charge of 2.0 μC is at the centre of a cubic Gaussian surface 9.0 cm on edge. What is the net electric flux through the surface?
A point charge causes an electric flux of −1.0 × 103 Nm2/C to pass through a spherical Gaussian surface of 10.0 cm radius centred on the charge.
- If the radius of the Gaussian surface were doubled, how much flux would pass through the surface?
- What is the value of the point charge?
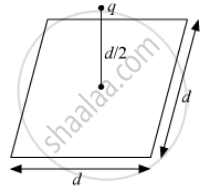
A point charge q is at a distance of d/2 directly above the centre of a square of side d, as shown the figure. Use Gauss' law to obtain the expression for the electric flux through the square.
Draw a graph to show the variation of E with perpendicular distance r from the line of charge.
A closed surface in vacuum encloses charges –q and +3q. The total electric flux emerging out of the surface is :
A spherical ball contracts in volume by 0.02% when subjected to a pressure of 100 atmosphere. Assuming one atmosphere = 105 Nm−2, the bulk modulus of the material of the ball is:
The electric field inside a spherical shell of uniform surface charge density is ______.
What is the nature of the Gaussian surface involved in the Gauss law of electrostatics?
