Advertisements
Advertisements
Question
For the polynomial `((x^3 + 2x + 1))/5 - 7/2 x^2 - x^6`, write the constant term
Solution
The given polynomial is `((x^3 + 2x + 1))/5 - 7/2 x^2 - x^6`
Since the given equation can be written as,
`((x^3 + 2x + 1))/5 - 7/2 x^2 - x^6`
= `1/5 x^3 + 2/5 x + 1/5 - 7/2 x^2 - x^6`
The constant term in the given polynomial is `1/5` as it has no variable x associated with it.
APPEARS IN
RELATED QUESTIONS
A plane left 30 minutes late than its scheduled time and in order to reach the destination 1500 km away in time, it had to increase its speed by 100 km/h from the usual speed. Find its usual speed.
Classify the following polynomials as linear, quadratic, cubic and biquadratic polynomials
`7t^4+4t^3+3t-2`
Define the zero of a polynomial.
The graph of a polynomial y = f(x), shown in Fig. 2.18. Find the number of real zeros of f(x).
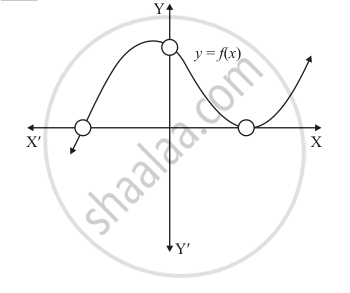
If a − b, a and b are zeros of the polynomial f(x) = 2x3 − 6x2 + 5x − 7, write the value of a.
State whether the given algebraic expression are polynomial? Justify.
`y + 1/y`
Divide. Write the quotient and the remainder.
40a3 ÷ (−10a)
Divide. Write the quotient and the remainder.
(5x3 − 3x2) ÷ x2
An asana is a body posture, originally and still a general term for a sitting meditation pose, and later extended in hatha yoga and modern yoga as exercise, to any type of pose or position, adding reclining, standing, inverted, twisting, and balancing poses. In the figure, one can observe that poses can be related to representation of quadratic polynomial.


The shape of the poses shown is:
Classify the following as a constant, linear, quadratic and cubic polynomials:
2 + x
