If a number is tripled and the result is increased by 5, we get 50. Find the number. - Mathematics

Question

If a number is tripled and the result is increased by 5, we get 50. Find the number.

Sum

Solution

Let the required number be x.
According to the question, the number is tripled and 5 is added to it
∴ 3x + 5
or, 3x + 5 = 50
or, 3x + 5 − 5 = 50 − 5 [Subtracting 5 from both the sides]
or, 3x = 45
or, `(3x)/3=45/3` [Dividing both the sides by 3]
or, x = 15
Thus, the required number is 15.

shaalaa.com

Use of Variables in Common Rules

Is there an error in this question or solution?

Q 6 Q 5 Q 7

Chapter 9: Linear Equation in One Variable - Exercise 9C [Page 144]

APPEARS IN

RS Aggarwal Mathematics [English] Class 6

Chapter 9 Linear Equation in One Variable
Exercise 9C | Q 6 | Page 144

Video TutorialsVIEW ALL [1]

view
Video Tutorials For All Subjects
Use of Variables in Common Rules

video tutorial00:54:47

RELATED QUESTIONS

Observe the patterns of digits made from line segments of equal length. You will find such segmented digits on the display of electronic watches or calculators.

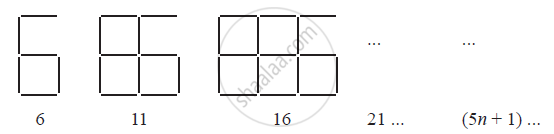
If the number of digits formed is taken to be n, the number of segments required to
form n digits is given by the algebraic expression appearing on the right of each pattern.

How many segments are required to form 5, 10, 100 digits of the kind −

Use the given algebraic expression to complete the table of number patterns.

S. No	Expression	Terms
S. No	Expression	1^st	2^nd	3^rd	4^th	5^th	...	10^th	...	100^th	...
1	2n - 1	1	3	5	7	9	-	19	-	-	-
2	3n + 2	5	8	11	14	-	-	-	-	-	-
3	4n + 1	5	9	13	17	-	-	-	-	-	-
4	7n + 20	27	34	41	48	-	-	-	-	-	-
5	n2 + 1	2	5	10	17	-	-	-	-	10001	-

The sum of three consecutive natural numbers is 114. Find the numbers.

Find two numbers such that one of them is five times the other and their difference is 132.

The area of a square having each side x is ______.

If I spend f rupees from 100 rupees, the money left with me is ______ rupees.

A cube is a three-dimensional figure as shown in the given figure. It has six faces and all of them are identical squares. The length of an edge of the cube is given by l. Find the formula for the total length of the edges of a cube.