Advertisements
Advertisements
Question
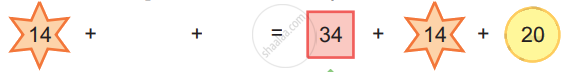
If AB + 7C = 102, where B ≠ 0, C ≠ 0, then A + B + C = 14.
Options
True
False
Solution
This statement is True.
Explanation:
AB
We have, + 7C
102
Here, B + C is either 2 or a two-digit number whose one's digit is 2.
If B = C = 1, lf 8 = 5, C = 7, A = 2 and A + B + C = 2 + 5 + 7 = 14
APPEARS IN
RELATED QUESTIONS
Do you remember magic triangles? Come now, let s make some magic squares.
- Fill this square using all the numbers from 21 to 29.
Rule: The total of each side is 75.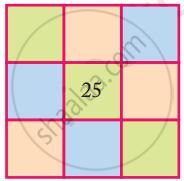
Use the same rule to fill the hexagons below.
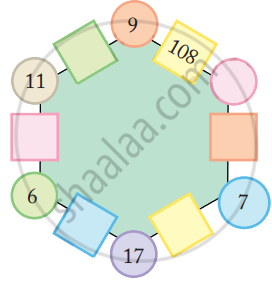
Now you also make your own magic hexagons.
Use the same rule to fill the hexagons below.
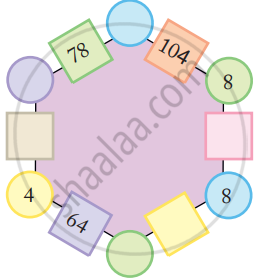
Now you also make your own magic hexagons.
Now you try and change these numbers into special numbers —
132
If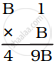 
then B = ______.
If a 3-digit number abc is divisible by 11, then ______ is either 0 or multiple of 11.
Number 7N + 1 will leave remainder 1 when divided by 7.
If N ÷ 5 leaves remainder 3 and N ÷ 2 leaves remainder 0, then N ÷ 10 leaves remainder 4.
Find the least value that must be given to number a so that the number 91876a2 is divisible by 8.
Fill in the blank space in the same way.
