Advertisements
Advertisements
Question
If from a two-digit number, we subtract the number formed by reversing its digits then the result so obtained is a perfect cube. How many such numbers are possible? Write all of them.
Solution
Let ab be any two-digit number.
Then, the digit formed by reversing it digits is ba.
Now, ab – ba = (10a + b) – (10b + a)
= (10a – a) + (b – 10b)
= 9a – 9b
= 9(a – b)
Further, since ab – ba is a perfect cube and is a multiple of 9.
∴ The possible value of a – b is 3.
i.e. a = b + 3
Here, b can take value from 0 to 6.
Hence, possible numbers are as follow.
For b = 0, a = 3, i.e. 30
For b = 1, a = 4, i.e. 41
For b = 2, a = 5, i.e. 52
For b = 3, a = 6, i.e. 63
For b = 4, a = 7, i.e. 74
For b = 5, a = 8, i.e. 85
For b = 6, a = 9, i.e. 96
APPEARS IN
RELATED QUESTIONS
Find the values of the letters in the following and give reasons for the steps involved.
A B
x 5
-------
C A B
-------
If 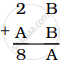 then A = ______ and B = ______.
then A = ______ and B = ______.
Find the value of the letters in the following question.
A A
+A A
XA Z
Find the value of the letters in the following question.
8 5
+ 4 A
B C 3
Find the value of the letters in the following question.
1 B A
+ A B A
8 B 2
Find the value of the letters in the following question.
A A
× A
C A B
Find the value of the letters in the following question.
A B
– B 7
4 5
If 2A7 ÷ A = 33, then find the value of A.
Work out the following multiplication.
12345679
× 9
Use the result to answer the following question.
By what number should 12345679 be multiplied to get 888888888?
Work out the following multiplication.
12345679
× 9
Use the result to answer the following question.
By what number should 12345679 be multiplied to get 999999999?
