Advertisements
Advertisements
Question
In a circle with radius 13 cm, two equal chords are at a distance of 5 cm from the centre. Find the lengths of the chords.
Sum
Solution
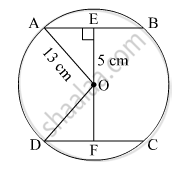
Let, O be the center of the circle and seg AB and seg CD are equal-length chords of the circle.
seg OE ⊥ seg AB such that, A-E-B and seg OF ⊥ seg CD such that, C-F-D.
OA = OD = 13 cm ...(Radius of the circle is 13 cm.)
In ∆AEO, by Pythagoras theorem,
OA2 = OE2 + EA2
∴ 132 = 52 + EA2
∴ EA2 = 169 – 25
∴ EA2 = 144
∴ EA = `sqrt(144)`
∴ EA = 12 cm
∴ EA = `1/2` BA ...(The perpendicular drawn from the center of the circle to the chord bisects the chord.)
∴ 12 = `1/2` BA
∴ BA = `12 xx 2`
∴ BA = 24 cm
chord AB ≅ chord CD
∴ CD = 24 cm
shaalaa.com
Properties of Congruent Chords
Is there an error in this question or solution?
