Advertisements
Advertisements
Question
In a coil of resistance 100 Ω a current is induced by changing the magnetic flux through it. The variation of current with time is shown in the figure. The magnitude of change in flux through the coil is ______.
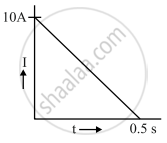
Options
200 Wb
275 Wb
225 Wb
250 Wb
Solution
In a coil of resistance 100 Ω a current is induced by changing the magnetic flux through it. The variation of current with time is shown in the figure. The magnitude of change in flux through the coil is 250 Wb.
Explanation:
e = `(ΔPhi)/(Δt), I = 1/R(ΔPhi)/(Δt)`
I Δt = `(ΔPhi)/R` = Area under l - t graph, R = 100 ohm
∴ ΔΦ = `100 xx 1/2 xx 10 xx 0.5 = 250` Wb
APPEARS IN
RELATED QUESTIONS
A rectangular coil having 60 turns and area of 0.4m2 is held at right angles to a uniform magnetic field of flux density 5 × 10-5T. Calculate the magnetic flux passing through it.
An inductor is connected to a battery through a switch. Explain why the emf induced in the inductor is much larger when the switch is opened as compared to the emf induced when the switch is closed.
Calculate magnetic flux density of the magnetic field at the centre of a circular coil of 50 turns, having a radius of 0.5m and carrying a current of 5 A.
Whenever the magnetic flux linked with an electric circuit changes, an emf is induced in the circuit. This is called ______.
The magnetic flux linked with a coil of N turns of area of cross-section A held with its plane parallel to the field B is ______.
The magnetic flux linked with the coil (in Weber) is given by the equation- Փ = 5t2 + 3t + 16. The induced EMF in the coil at time, t = 4 will be ______.
The dimensions of magnetic flux are ______
The unit of magnetic flux in SI is ______
A square of side L meters lies in the x-y plane in a region, where the magnetic field is given by `B = Bo(2hati + 3hatj + 4hatk)`T, where B0 is constant. The magnitude of flux passing through the square is ______.
A circular coil of 1000 turns each with area 1 m2 is rotated about its vertical diameter at the rate of one revolution per second in a uniform horizontal magnetic field of 0.07T. The maximum voltage generation will be ______ V.
