Advertisements
Advertisements
Question
In a right circular cone, height, radius and slant height do not always be sides of a right triangle.
Options
True
False
Solution
This statement is False.
Explanation:
On rotating a right-angled triangle AOB about OA, it generates a cone. The point A is the vertex of a cone. Its base is a circle with centre O and radius OB. The length OA is the height of the cone and the length AB is called its slant height.
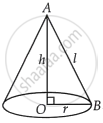
Clearly, ∠AOB = 90°
Let the radius of the base be r units, height = h units and slant height = l units, then
`l^2 = h^2 + r^2 \implies l = sqrt(h^2 + r^2)`
Hence, in right circular cone, height, radius and slant height always be sides of a right triangle.
APPEARS IN
RELATED QUESTIONS
If the volume of a right circular cone of height 9 cm is 48 `pi` cm3, find the diameter of its base.
If the radius and slant height of a cone are in the ratio 7 : 13 and its curved surface area is 286 cm2, find its radius.
The total surface area of a cone of radius `r/2` and length 2l, is
If the volume of two cones are in the ratio 1 : 4 and their diameters are in the ratio 4 : 5, then the ratio of their heights, is
If the height and radius of a cone of volume V are doubled, then the volume of the cone, is
A right circular cylinder and a right circular cone have the same radius and the same volume. The ratio of the height of the cylinder to that of the cone is
The diameters of two cones are equal. If their slant heights are in the ratio 5 : 4, the ratio of their curved surface areas, is
If the heights of two cones are in the ratio of 1 : 4 and the radii of their bases are in the ratio 4 : 1, then the ratio of their volumes is
There are 25 persons in a tent which is conical in shape. Every person needs an area of 4 sq.m. of the ground inside the tent. If height of the tent is 18 m, find the volume of the tent.
If the ratio of radius of base and height of a cone is 5:12 and its volume is 314 cubic metre. Find its perpendicular height and slant height. (π = 3.14)
